Bernstein method
method of auxiliary functions
A method which is employed in the theory of linear and non-linear partial differential equations. Bernstein's method consists in introducing certain new (auxiliary) functions, which depend on the solution being sought, and which make it possible to establish a priori estimates of the maximum modulus of the derivatives of this solution of the required order.
A simple example of the application of Bernstein's method is the a priori estimate of the modulus of the derivatives of the solution of the Dirichlet problem for the non-linear (quasi-linear) elliptic equation
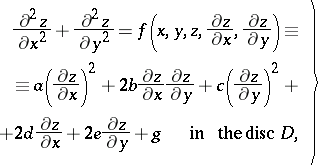 | (*) |
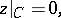 |
where  are smooth functions of
are smooth functions of 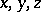 ;
; 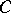 is the circle, the boundary of the disc
is the circle, the boundary of the disc 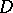 with radius
with radius 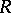 (the assumption to the effect that
(the assumption to the effect that 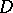 is a disc and
is a disc and 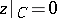 is immaterial, since the general case of an arbitrary-connected domain and inhomogeneous boundary condition is readily reduced to the case under consideration by a change of the function and a conformal transformation of the domain).
is immaterial, since the general case of an arbitrary-connected domain and inhomogeneous boundary condition is readily reduced to the case under consideration by a change of the function and a conformal transformation of the domain).
If 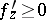 , then the estimated maximum modulus
, then the estimated maximum modulus 
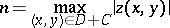 |
of the solution of problem (*) is immediately obtained from the maximum principle.
In order to prove that a regular solution of problem (*) exists it is sufficient to have a priori estimates of the maximum modulus of the derivatives of the solution up to the third order (cf. Continuation method (to a parametrized family)). To estimate 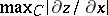 and
and 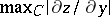 , it is sufficient to estimate
, it is sufficient to estimate 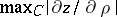 (since
(since 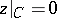 ), where
), where 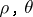 are polar coordinates in the disc
are polar coordinates in the disc 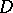 . Now introduce a new (auxiliary) function
. Now introduce a new (auxiliary) function  , given by the formula
, given by the formula
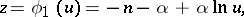 |
where 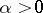 will be selected later. The function
will be selected later. The function 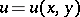 varies from
varies from  to
to 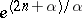 in the same direction as
in the same direction as 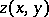 (
(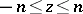 ). Since
). Since
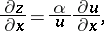 |
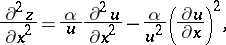 |
and similar results for derivatives with respect to  , it follows that
, it follows that  satisfies the equation
satisfies the equation
 |
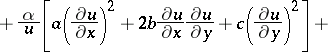 |
 |
Let  be the upper bound of
be the upper bound of 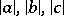 in
in  , and let
, and let 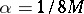 . If
. If 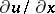 and
and 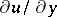 are considered as current coordinates in the plane, and
are considered as current coordinates in the plane, and 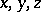 as parameters, the equation
as parameters, the equation 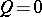 is the equation of an ellipse, since the determinant
is the equation of an ellipse, since the determinant 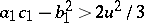 , where
, where
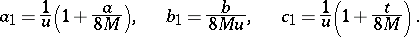 |
Thus, for any 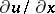 and
and 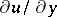 ,
, 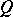 will not be smaller than a certain negative number
will not be smaller than a certain negative number  ,
, 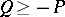 (the number
(the number 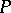 is readily obtained in explicit form). If one introduces the function
is readily obtained in explicit form). If one introduces the function 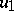 given by the formula
given by the formula
 |
one obtains
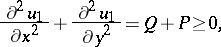 |
and 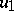 attains its maximum on the boundary
attains its maximum on the boundary  of the domain
of the domain  and, since
and, since 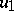 is constant on
is constant on  , one has
, one has
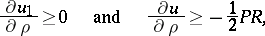 |
where 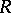 is the radius of the circle
is the radius of the circle 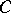 . Hence it is possible to find a negative lower bound for
. Hence it is possible to find a negative lower bound for 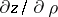 :
:
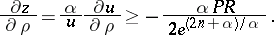 |
If the same reasoning is applied to a second auxiliary function 
 |
one obtains an estimate from above
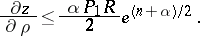 |
Thus, 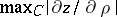 is estimated, which means that
is estimated, which means that 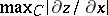 and
and 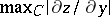 are estimated as well. The estimate of the maximum modulus of the first derivatives inside the domain
are estimated as well. The estimate of the maximum modulus of the first derivatives inside the domain 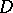 is performed in a similar manner: introduce an auxiliary function
is performed in a similar manner: introduce an auxiliary function  given by the formula
given by the formula
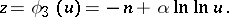 |
The function  varies in the same direction as
varies in the same direction as  , from
, from  to
to 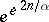 . In view of (*), on may write the following expression for
. In view of (*), on may write the following expression for 
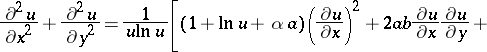 |
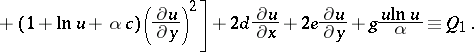 |
Considerations similar to those given above show that if the function
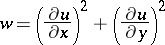 |
attains a maximum in the domain  , this maximum does not exceed some number, the value of which depends solely on
, this maximum does not exceed some number, the value of which depends solely on  and
and 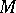 . This yields the required estimates of
. This yields the required estimates of 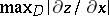 and
and 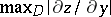 .
.
Bernstein's method may also be used to estimate, in a similar manner, the maximum modulus in the domain 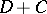 of all highest derivatives of the solution (the only other operation which is required is the differentiation of the initial equation).
of all highest derivatives of the solution (the only other operation which is required is the differentiation of the initial equation).
The method was first utilized by S.N. Bernstein [e theory of functions','../c/c025430.htm','Continuation method (to a parametrized family)','../c/c025520.htm','Euler–Lagrange equation','../e/e036510.htm','Fourier series','../f/f041090.htm','Functions of a real variable, theory of','../f/f042130.htm','Hilbert problems','../h/h120080.htm','Jackson inequality','../j/j054000.htm','Laplace theorem','../l/l057530.htm','Lebesgue constants','../l/l057800.htm','Limit theorems','../l/l058920.htm','Linear elliptic partial differential equation and system','../l/l059180.htm','Lyapunov theorem','../l/l061200.htm','Mathematical statistics','../m/m062710.htm','Minimal surface','../m/m063920.htm','Markov–Bernstein-type inequalities','../m/m110060.htm','Ornstein–Uhlenbeck process','../o/o070240.htm','Orthogonal polynomials','../o/o070340.htm','Plateau problem, multi-dimensional','../p/p072850.htm','Quasi-analytic class','../q/q076370.htm')" style="background-color:yellow;">S.N. Bernshtein] . The method was subsequently extended and was systematically utilized in the study of various problems for elliptic and parabolic differential operators [3], [4], [5].
References
| [1a] | S.N. [S.N. Bernshtein] Bernstein, "Sur la généralisation du problème de Dirichlet (première partie)" Math. Ann. , 62 (1906) pp. 253–271 |
| [1b] | S.N. [S.N. Bernshtein] Bernstein, "Sur la généralisation du problème de Dirichlet (deuxième partie)" Math. Ann. , 69 (1910) pp. 82–136 |
| [2] | S.N. Bernshtein, , Collected works , 3 , Moscow (1960) |
| [3] | O.A. Ladyzhenskaya, N.N. Ural'tseva, "Linear and quasilinear elliptic equations" , Acad. Press (1968) (Translated from Russian) |
| [4] | A.V. Pogorelov, "Die Verbiegung konvexer Flächen" , Akademie Verlag (1957) (Translated from Russian) |
| [5] | O.A. Oleinik, S.N. Kruzhkov, "Quasi-linear parabolic equations of second order in several independent variables" Russian Math. Surveys , 16 : 2 (1961) pp. 105–146 Uspekhi Mat. Nauk , 16 : 5 (1961) pp. 115–155 |
Bernstein method. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bernstein_method&oldid=13167