Bernstein-Baskakov-Kantorovich operator
To give a simpler proof of the Weierstrass approximation theorem (cf. Weierstrass theorem), S.N. Bernstein [a3] defined a new sequence of linear positive operators defined for functions continuous on  as
as
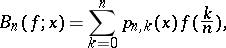 |
where
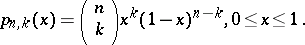 |
This sequence turned out to be a very interesting sequence, easy to deal with and having many applications in mathematical analysis. It has been extensively studied by G.G. Lorentz in [a13]. It is also of great interest in approximation theory, and as a result of that L.V. Kantorovich [a12] devised a modification of these operators, known as the Bernstein–Kantorovich polynomials; they are defined over a larger class of functions, e.g.,  ,
, 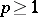 . The operator is defined as
. The operator is defined as
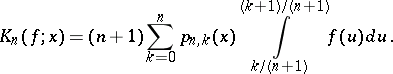 |
The approximation properties of this sequence have been discussed in detail (cf., e.g., [a7], [a14]).
Another interesting modification of the Bernstein polynomials was introduced by J.L. Durrmeyer [a8] for approximating functions in  ,
,  . This sequence is given by
. This sequence is given by
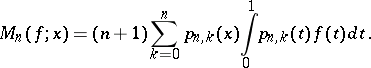 |
M.M. Derriennic [a5] has studied approximation properties of the operators  . Subsequently, important contributions to the study of these operators were made (see, e.g., [a6], [a9]). Modifications similar to that of Durrmeyer have been studied for other sequences of operators as well, e.g., for Szász, Lupas, and Baskakov operators (cf., e.g., [a10], [a11], [a17]).
. Subsequently, important contributions to the study of these operators were made (see, e.g., [a6], [a9]). Modifications similar to that of Durrmeyer have been studied for other sequences of operators as well, e.g., for Szász, Lupas, and Baskakov operators (cf., e.g., [a10], [a11], [a17]).
It has been observed that the order of approximation by these sequences of linear positive operators increases with the smoothness of the function. E. Voronovskaya [a20] has shown that the order of approximation by Bernstein polynomials cannot be improved beyond 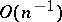 . This phenomenon is true for every other sequence of linear operators. The first attempt at somehow improving the order of approximation was made by P.L. Butzer [a4], who showed that by taking a linear combination of the Bernstein polynomials the order of approximation considerably improves for smoother functions. More general combinations have been studied for other sequences of operators (cf., e.g., [a15]). There is still another approach towards improving the order of approximation, by taking iterative combinations (cf., e.g., [a16]).
. This phenomenon is true for every other sequence of linear operators. The first attempt at somehow improving the order of approximation was made by P.L. Butzer [a4], who showed that by taking a linear combination of the Bernstein polynomials the order of approximation considerably improves for smoother functions. More general combinations have been studied for other sequences of operators (cf., e.g., [a15]). There is still another approach towards improving the order of approximation, by taking iterative combinations (cf., e.g., [a16]).
Another phenomenon of interest is the study of simultaneous approximation (approximation of derivatives of a function by the derivatives of corresponding order of operators). The first remarkable result in this direction is due to Lorentz [a13]. For further research in this area, see [a5], [a6], [a9], [a11], [a17].
V.A. Baskakov [a1] gave a sequence of linear positive operators which includes the Bernstein polynomials, the Szász operators, the Lupas operators, etc., as particular cases. The sequence is defined by
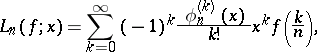 |
where  is a family of real-valued functions such that:
is a family of real-valued functions such that:
i) 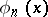 can be expanded in a Taylor series in
can be expanded in a Taylor series in 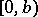 (where
(where 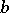 may be equal to
may be equal to 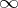 );
);
ii)  ;
;
iii)  ,
, 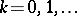 , for
, for  ;
;
iv) 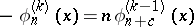 ,
, 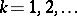 ,
, 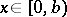 , for some constant
, for some constant  ;
;
v) for any fixed constant 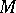 ,
,  for
for  . Studies similar to those for the Bernstein polynomials have been carried out for the Baskakov operators (cf., e.g., [a10], [a11], [a15]).
. Studies similar to those for the Bernstein polynomials have been carried out for the Baskakov operators (cf., e.g., [a10], [a11], [a15]).
The converse problem of inferring the nature of smoothness of a function from its order of approximation led to the study of inverse and saturation theorems for the above sequences of operators. Such problems have been studied both locally as well as globally.
Some important references for the study of such problems are [a2], [a6], [a7], [a9], [a15], [a16], [a18], [a19].
References
| [a1] | V.A. Baskakov, "An example of a sequence of linear positive operators in the space of continuous functions" Dokl. Akad. Nauk SSSR , 113 (1957) pp. 249–251 (In Russian) |
| [a2] | M. Becker, R.J. Nessel, "An elementary approach to inverse approximation theorems" J. Approx. Th. , 23 (1978) pp. 99–103 |
| [a3] | S.N. Bernstein, "Démonstration du théorème de Weierstrass, fondée sur le calcul des probabilités" Commun. Soc. Math. Kharkow (2) , 13 (1912–13) pp. 1–2 |
| [a4] | P.L. Butzer, "Linear combinations of Bernstein polynomials" Canad. J. Math. , 5 (1953) pp. 559–567 |
| [a5] | M.M. Derriennic, "Sur l'approximation de fonctions intégrable sur 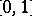 par des polynômes de Bernstein modifiés" J. Approx. Th. , 31 (1981) pp. 325–343 par des polynômes de Bernstein modifiés" J. Approx. Th. , 31 (1981) pp. 325–343 |
| [a6] | Z. Ditzian, K. Ivanov, "Bernstein-type operators and their derivatives" J. Approx. Th. , 56 (1989) pp. 72–90 |
| [a7] | Z. Ditzian, C.P. May, "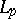 -saturation and inverse theorems for modified Bernstein polynomials" Indiana Univ. Math. J. , 25 (1976) pp. 733–751 -saturation and inverse theorems for modified Bernstein polynomials" Indiana Univ. Math. J. , 25 (1976) pp. 733–751 |
| [a8] | J.L. Durrmeyer, "Une formule d'inversion de la transformée de Laplace: Applications à la théorie des moments" , Fac. Sci. l'Univ. Paris (1967) (Thèse de 3e cycle) |
| [a9] | H.H. Gonska, Xin-Long Zhou, "A global inverse theorem on simultaneous approximation by Bernstein–Durrmeyer operators" J. Approx. Th. , 67 (1991) pp. 284–302 |
| [a10] | M. Heilmann, "Approximation auf  durch das Verfahren der Operatoren vom Baskakov–Burrmeyer Typ" , Univ. Dortmund (1987) (Dissertation) durch das Verfahren der Operatoren vom Baskakov–Burrmeyer Typ" , Univ. Dortmund (1987) (Dissertation) |
| [a11] | M. Heilmann, M.W. Müller, "On simultaneous approximation by the method of Baskakov–Durrmeyer operators" Numer. Funct. Anal. Optim. , 10 (1989) pp. 127–138 |
| [a12] | L.V. Kantorovich, "Sur certaines developments suivant les polynômes de la forme de S. Bernstein I-- II" C.R. Acad. Sci. USSR A (1930) pp. 563–568; 595–600 |
| [a13] | G.G. Lorentz, "Bernstein polynomials" , Univ. Toronto Press (1953) |
| [a14] | V. Maier, "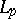 -approximation by Kantorovic operators" Anal. Math. , 4 (1978) pp. 289–295 -approximation by Kantorovic operators" Anal. Math. , 4 (1978) pp. 289–295 |
| [a15] | C.P. May, "Saturation and inverse theorems for combinations of a class of exponential-type operators" Canad. J. Math. , 28 (1976) pp. 1224–1250 |
| [a16] | C.A. Micchelli, "The saturation class and iterates of the Bernstein polynomials" J. Approx. Th. , 8 (1973) pp. 1–18 |
| [a17] | R.P. Sinha, P.N. Agrawal, V. Gupta, "On simultaneous approximation by modified Baskakov operators" Bull. Soc. Math. Belg. B , 43 (1991) pp. 217–231 |
| [a18] | Y. Suzuki, "Saturation of local approximation by linear positive operators of Bernstein type" Tôhoku Math. J. , 19 (1967) pp. 429–453 |
| [a19] | Y. Suzuki, S. Watanabe, "Some remarks on saturation problems in the local approximation II" Tôhoku Math. J. , 21 (1969) pp. 65–83 |
| [a20] | E. Voronowskaja, "Détermination de la forme asymptotique d'approximation des fonctions par les polynômes de S. Bernstein" C.R. Acad. Sci. USSR (1932) pp. 79–85 |
Bernstein-Baskakov-Kantorovich operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bernstein-Baskakov-Kantorovich_operator&oldid=12902