Additive theory of ideals
A branch of modern algebra. Its principal task is to represent any ideal of a ring (or of another algebraic system) as the intersection of a finite number of ideals of special type (primary, tertiary, primal, uniserial, etc.). The type of the representation is so chosen that: 1) for any ideal there exists a representation, in other words, some "existence" theorem holds; 2) the representations chosen must be unique apart from certain limitations or, in other words, some "uniqueness" theorem must hold. The fundamental principles of the additive theory of ideals were introduced in the 1920s and the 1930s by E. Noether [1] and W. Krull [2].
All special features of the additive theory of ideals are clearly manifested in the case of rings. Let  be a Noetherian ring, i.e. an associative ring with the maximum condition for ideals. If
be a Noetherian ring, i.e. an associative ring with the maximum condition for ideals. If  is an ideal of
is an ideal of  , then there exists a largest ideal
, then there exists a largest ideal  of
of 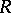 for which
for which  for some integer
for some integer  . This ideal
. This ideal  is known as the primary radical of
is known as the primary radical of  (in
(in  ) and is denoted by
) and is denoted by  . An ideal
. An ideal 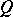 of
of 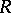 is said to be primary if for any two ideals
is said to be primary if for any two ideals  and
and 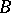 of
of 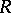 , the condition
, the condition
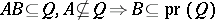 |
is satisfied. The intersection theorem is valid for primary ideals: The intersection of two primary ideals having the same primary radical 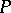 is itself a primary ideal with radical
is itself a primary ideal with radical 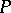 . This theorem is used to prove an existence theorem: If the ring
. This theorem is used to prove an existence theorem: If the ring  is commutative, then for any ideal
is commutative, then for any ideal 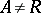 there exists a representation of
there exists a representation of 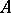 as the intersection of a finite number of primary ideals
as the intersection of a finite number of primary ideals  :
:
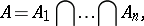 | (1) |
such that none of the ideals 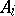 contains the intersection of the other ones, and such that the primary radicals
contains the intersection of the other ones, and such that the primary radicals 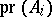 are pairwise different. Such representations are known as non-contractible or primarily reduced [1], [4]. The uniqueness theorem holds for such representations: If (1) holds and
are pairwise different. Such representations are known as non-contractible or primarily reduced [1], [4]. The uniqueness theorem holds for such representations: If (1) holds and
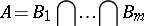 | (2) |
is a second primarily-reduced representation of the ideal 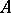 of the ring
of the ring 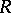 , then
, then  and
and 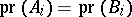 for
for 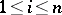 , provided the ideals
, provided the ideals 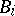 are suitably renumbered.
are suitably renumbered.
The additive theory of ideals of Noetherian commutative rings (the classical additive theory of ideals) has found numerous applications in various branches of mathematics.
If the ring  is non-commutative, the above-mentioned existence theorem is no longer valid, but the uniqueness and intersection theorems still hold. This is why, ever since the 1930s, repeated attempts have been made to find a generalization of classical primarity to the non-commutative case such that the existence theorem, too, remains valid. Such a generalization has in fact been found [4], and is known as tertiarity (cf. Tertiary ideal). It was subsequently shown that, within certain natural limitations, tertiarity is the only "good" generalization of the concept of primarity [6], [7], [8].
is non-commutative, the above-mentioned existence theorem is no longer valid, but the uniqueness and intersection theorems still hold. This is why, ever since the 1930s, repeated attempts have been made to find a generalization of classical primarity to the non-commutative case such that the existence theorem, too, remains valid. Such a generalization has in fact been found [4], and is known as tertiarity (cf. Tertiary ideal). It was subsequently shown that, within certain natural limitations, tertiarity is the only "good" generalization of the concept of primarity [6], [7], [8].
During the 1960s the additive theory of ideals developed further within the framework of lattice theory, of systems with fractions and of multiplicative systems [4], [5], [6]; this stimulated the development of the additive theory of ideals for non-associative rings, normal divisors of a group and submodules of a module.
References
| [1] | E. Noether, "Idealtheorie in Ringbereichen" Math. Ann. , 83 (1921) pp. 24–66 |
| [2] | W. Krull, "Idealtheorie in Ringen ohne Endlichkeitsbedingung" Math. Ann. , 101 (1929) pp. 729–744 |
| [3] | O. Zariski, P. Samuel, "Commutative algebra" , 1 , Springer (1975) |
| [4] | L. Lesieur, R. Croisot, "Algèbre noethérienne noncommutative" , Gauthier-Villars (1963) |
| [5] | K. Murata, "Additive ideal theory in multiplicative systems" J. Inst. Polytechn. Osaka City Univ. (A) , 10 : 2 (1959) pp. 91–115 |
| [6] | V.A. Andrunakievich, Yu.M. Ryabukhin, "The additive theory of ideals in systems with residuals" Math. USSR-Izv. , 1 : 5 (1967) pp. 1011–1040 Izv. Akad. Nauk SSSR Ser. Mat. , 31 (1967) pp. 1057–1090 |
| [7] | J.A. Riley, "Axiomatic primary and tertiary decomposition theory" Trans. Amer. Math. Soc. , 105 (1962) pp. 177–201 |
| [8] | I.M. Goyan, Yu.M. Ryabukhin, "On the axiomatic additive theory of Riley ideals" Mat. Issl. , 2 : 1 (1967) pp. 14–25 (In Russian) |
| [9] | L. Fuchs, "On primal ideals" Proc. Amer. Math. Soc. , 1 (1950) pp. 1–6 |
| [10] | Itogi Nauk. Algebra Topol. Geom. 1965 (1967) pp. 133–180 |
Comments
A primary representation is also called a primary decomposition. More generally one has primary decompositions for submodules 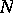 of modules
of modules  over a Noetherian ring
over a Noetherian ring 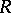 . This is a representation
. This is a representation
 |
where each  consists of a single prime ideal
consists of a single prime ideal  . (By definition
. (By definition  for a module
for a module 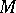 , the set of prime ideals associated to
, the set of prime ideals associated to 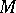 , is the collection of all prime ideals
, is the collection of all prime ideals 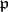 for which there exists an
for which there exists an 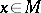 such that
such that  ). There is also a corresponding uniqueness theorem, stating that there is a reduced decomposition, which of course means that
). There is also a corresponding uniqueness theorem, stating that there is a reduced decomposition, which of course means that 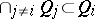 holds for no
holds for no  and that the
and that the 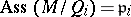 are all different.
are all different.
References
| [a1] | N. Bourbaki, "Algèbre commutative" , Hermann (1961) pp. Chapt. 3; 4 |
Additive theory of ideals. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Additive_theory_of_ideals&oldid=18106