Whitney mapping

Let be a compact Hausdorff space. The hyperspace of is denoted by ; the subspace of , consisting of all sub-continua of is denoted by . A Whitney mapping of is a real-valued continuous function on (or on 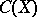) with the following properties:

1) 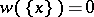for each ;

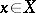2) if 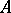and 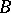are in the domain of and if 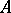is a proper subset of , then .

A set of type 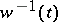, for , is called a Whitney level.

The existence of a Whitney function on implies that is a 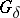-subset of its hyperspace, which in turn implies that is metrizable (cf. also Metrizable space). One of the simplest constructions of a Whitney mapping for a compact metrizable space 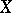is as follows. Let be a countable open base of 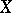, and, for each pair 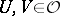such that 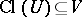, fix a mapping 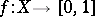which equals on and outside 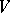. Enumerate these functions as 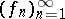and let

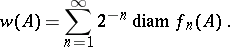Below it is assumed that all spaces are metric continua. Whitney functions have been developed as a fundamental tool in continua theory. Their first use in this context was involved with order arcs in 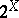, and led to a proof that 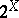is acyclic and that both and 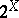are even contractible if 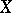is Peanian (1942). Later on they became a subject of study in their own right.

A Whitney property is a topological property 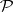such that if a metric continuum has 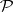, then so does each Whitney level of it in . Examples are: being a (locally connected) continuum; being a hereditarily indecomposable continuum; being a (pseudo-) arc or a circle; etc. Counterexamples are: being contractible; being acyclic in Alexander–Čech cohomology; being homogeneous; being a Hilbert cube.

Whitney functions were introduced by H. Whitney [a4] in 1933 in a different context. They were first used by J.L. Kelley [a2] to study hyperspaces. Every metric continuum can occur as a Whitney level [a1]. For an account of continua theory see [a3].

References

[a1]	W.J. Charatonik, "Continua as positive Whitney levels" Proc. Amer. Math. Soc. , 118 (1993) pp. 1351–1352
[a2]	J.L. Kelley, "Hyperspaces of a continuum" Trans. Amer. Math. Soc. , 52 (1942) pp. 22–36
[a3]	S.B. Nadler, "Hyperspaces of sets" , M. Dekker (1978)
[a4]	H. Whitney, "Regular families of curves" Ann. of Math. , 2 (1933) pp. 244–270