Scattered space
Scattered spaces have their origin in Cantors investigations into the uniqueness of trigonometric series. His theorem reads (in modern terminology): If the partial sums of a trigonometric series
 |
converge to zero except possibly on a set of points of finite scattered height, then all coefficients of the series must be zero.
Scattered spaces and their scattered height are defined as follows. One first defines for any space  a transfinite sequence
a transfinite sequence  of subspaces: Let
of subspaces: Let  , for any ordinal
, for any ordinal  , let
, let  be the derived set of
be the derived set of  , and if
, and if  is a limit ordinal, put
is a limit ordinal, put  .
.
There is a first ordinal  for which
for which  . If this derived set
. If this derived set  is empty, one calls
is empty, one calls  a scattered space and the ordinal
a scattered space and the ordinal  its scattered height.
its scattered height.
If  is compact, then it is readily seen that
is compact, then it is readily seen that 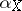 must be a successor ordinal, say
must be a successor ordinal, say  . The set
. The set  is finite. It is a classical result of S. Mazurkiewicz and J. Sierpiński that a countable compact scattered space
is finite. It is a classical result of S. Mazurkiewicz and J. Sierpiński that a countable compact scattered space  is determined completely by the ordinal
is determined completely by the ordinal 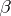 and the number
and the number  of points in
of points in  :
:  is homeomorphic to the set of ordinal numbers less than or equal to
is homeomorphic to the set of ordinal numbers less than or equal to  .
.
Compact scattered spaces correspond, via Stone duality, to the so-called superatomic Boolean algebras; these are defined to be those algebras for which every (non-trivial) homomorphic image has an atom. Because of this duality one may say that the structure of compact scattered spaces is understood best.
An important family of scattered spaces (of height  ) is constructed as follows: Take an infinite set
) is constructed as follows: Take an infinite set  and a family
and a family  of countably-infinite subsets that is almost disjoint, i.e., if
of countably-infinite subsets that is almost disjoint, i.e., if  , then
, then  is finite. The union
is finite. The union  is topologized by declaring
is topologized by declaring  to be an open discrete subspace and giving an element
to be an open discrete subspace and giving an element  of
of  basic neighbourhoods of the form
basic neighbourhoods of the form  , where
, where 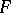 is a finite subset of
is a finite subset of  . By varying the family
. By varying the family  one can obtain various interesting examples of topological spaces, for example, in this way one can make a pseudo-compact space that is not countably compact (cf. Countably-compact space).
one can obtain various interesting examples of topological spaces, for example, in this way one can make a pseudo-compact space that is not countably compact (cf. Countably-compact space).
References
| [a1] | G. Cantor, "Über die Ausdehnung eines Satzes aus der Theorie der trigonometrischen Reihen" Math. Ann. , 5 (1872) pp. 123–132 Zbl 04.0101.02 |
| [a2] | S. Mŕowka, "On completely regular spaces" Fund. Math. , 41 (1954) pp. 105–106 Zbl 0055.41304 |
| [a3] | J. Roitman, "Superatomic Boolean algebras" J.D. Monk (ed.) R. Bonnet (ed.) , Handbook of Boolean algebras , 1–3 , North-Holland (1989) pp. Chapt. 19; pp. 719–740 Zbl 0671.06001 |
Scattered space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Scattered_space&oldid=42510