Linear algebraic groups, arithmetic theory of

The theory that studies arithmetic properties of linear algebraic groups (cf. Linear algebraic group), defined, as a rule, over a global field.

One of the principal objects of study in the arithmetic theory of linear algebraic groups are arithmetic subgroups of an algebraic group $G$ (see Arithmetic group), and one of the principal technical instruments is the adèle group $G_{\mathbf{A}}$. On $G_{\mathbf{A}}$ one can define in a natural way a measure, called the Tamagawa measure. One of the first questions that arises here is the following: When is the volume of the quotient space of $G_{\mathbf{A}}$ with respect to the principal adèle subgroup $G_k$ finite? A complete answer to this was obtained by A. Borel. It turned out that the volume of $G_{\mathbf{A}}/G_k$ is always finite for a semi-simple group. The solution of this problem preceded the construction of a reduction theory for arithmetic groups (see [5], [6]).

Using the theory of reduction for principal adèle subgroups, it was possible in many cases to calculate the volume of $G_{\mathbf{A}}/G_k$, which is called the Tamagawa number of the group $G$. For example, for an orthogonal group $G$ the Tamagawa number $\tau(G)$, and this is actually equivalent to a fundamental result in the analytic theory of quadratic forms (see [1]). The study of the structure of arithmetic groups (begun in [6]) was then extended in various directions. First of all one should mention investigations on the congruence problem, the problem of maximality of arithmetic subgroups and the problem of the genus of arithmetic groups.

In all basic questions in the arithmetic theory of linear algebraic groups an essential role is played by approximation theorems, which reduce the investigation of arithmetic properties of algebraic groups defined over global fields to the investigation of arithmetic properties of algebraic groups defined over local fields. Of greatest significance is the problem of strong approximation in algebraic groups, which consists of the following. Let $V = \{v\}$ be the set of all inequivalent norms of a field $k$, let $k_v$ be the completion of $k$ with respect to $v$, let $O_v$ be the ring of integral elements of $k_v$, and let $\mathfrak{P}_v$ be the maximal ideal of $O_v$. For an arbitrary finite subset $S \subset V$, let $G_S$ denote the subgroup of $G_{\mathbf{A}}$ in which all $v$-components with $v \notin S$ are equal to the identity. The question is: When is $\overline{G_S G_k} = G_{\mathbf{A}}$? (Here the bar denotes closure in the topology of $G_{\mathbf{A}}$.) If $S = \infty$ ($\infty$ is the set of all Archimedean norms of $k$), then an equivalent formulation of this problem is the following: For any $v_i \in S$, $i=1,\ldots,n$, any $a_{v_i} \in G_{k_{v_i}}$ and positive integers $m_i$, when does the system of congruences $$ x \equiv a_{v_i} \pmod{\mathfrak{P}_{v_i}^{m_i}} $$ where $x \in G_{O_v}$ for $v \in \cup \{v_1,\ldots,v_n\}$, have a solution in the group $G_k$?

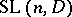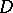M. Eichler [13] solved the problem of strong approximation for the groups where is a skew-field of finite -rank. Later, various special cases of this problem were investigated by M. Kneser, G. Shimura and A. Weil (see [4]). The problem of strong approximation has been solved (see [9], [10]) for classical groups over number fields, and necessary conditions have been found for its affirmative solution in the general case, namely: a) 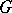must be simply connected as an algebraic group; and b) if 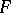is any simple component of the semi-simple part of , then must be non-compact. The necessity of these conditions has been proved [14] for a function field. Finally, the sufficiency of conditions a) and b) has been proved

(see also [16]) over both number fields and function fields, which gives a complete solution of the strong approximation problem. At the base of the method of proof lies the reduction of this problem to the proof of the Kneser–Tits hypothesis on the structure of simply-connected groups over local fields: If is a 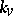-simple simply-connected 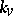-isotropic group, then 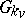is generated by unipotent elements, or, equivalently, the quotient group of 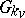with respect to its centre is simple in the abstract sense. As the simplest application of the strong approximation theorem one obtains the following fact: Suppose that 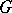has the strong approximation property with respect to 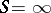and let 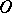be the ring of integral elements of 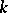, then

this shows that the arithmetic of 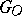is determined to a significant extent by the arithmetic of the local components .

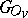Together with strong approximation, an important role in the arithmetic theory of linear algebraic groups is played by the property of weak approximation of an algebraic group 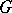with respect to 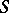, which consists of the fact that the image of 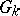under the canonical projection 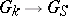is dense in 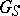. All simply-connected groups have the weak approximation property. On the other hand, there are examples of semi-simple groups and algebraic tori that do not have the weak approximation property (see [11], [2]). Nevertheless, for a wide class of non-simply-connected semi-simple groups, in particular, for adjoint groups, the weak approximation property is satisfied [12]. If 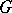is an algebraic torus and if for every 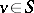the torus 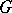splits over a cyclic extension of the field , then 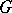has the weak approximation property with respect to 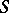. In certain cases this property is satisfied for algebraic groups over an arbitrary field (see [11]). 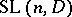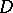There is a conjecture (see [11]) that the weak approximation property is satisfied for the groups where is a skew-field of finite 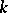-rank over an arbitrary infinite field 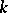. However, the development of reduced 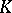-theory has led to a negative answer (see [15]): For the groups the deviation from weak approximation can be arbitrary large.

An important role in the arithmetic theory of linear algebraic groups is played by cohomology methods, in particular the Hasse principle (see Galois cohomology).

References

[1]	A. Borel (ed.) G.D. Mostow (ed.) , Algebraic groups and discontinuous subgroups , Proc. Symp. Pure Math. , 9 , Amer. Math. Soc. (1966) MR0202512 Zbl 0171.24105
[2]	J.W.S. Cassels (ed.) A. Fröhlich (ed.) , Algebraic number theory , Acad. Press (1986) MR0911121 Zbl 0645.12001 Zbl 0153.07403
[3]	A. Weil, "Basic number theory" , Springer (1974) MR0427267 Zbl 0326.12001
[4]	A. Weil, "Sur la formule de Siegel dans la théorie des groupes classiques" Acta Math. , 113 (1965) pp. 1–87 MR0223373 Zbl 0161.02304
[5]	A. Borel, "Arithmetic properties of linear algebraic groups" , Proc. Internat. Congress mathematicians (Stockholm, 1962) , Inst. Mittag-Leffler (1963) pp. 10–22 MR0175901 Zbl 0134.16502
[6]	A. Borel, Harish-Chandra, "Arithmetic subgroups of algebraic groups" Ann. of Math. , 75 (1962) pp. 485–535 MR0147566 Zbl 0107.14804
[7a]	V.P. Platonov, "The problem of strong approximation and the Kneser–Tits conjecture for algebraic groups" Math. USSR Izv. , 3 (1969) pp. 1139–1148 Izv. Akad. Nauk SSSR Ser. Mat. , 33 (1969) pp. 1211–1219 Zbl 0217.36301
[7b]	V.P. Platonov, "Addendum to "The problem of strong approximation and the Kneser–Tits conjecture for algebraic groups" " Math. USSR Izv. , 4 (1970) pp. 784–786 Izv. Akad. Nauk SSSR Ser. Mat. , 34 (1970) pp. 775–777 Zbl 0236.20034
[8]	V.P. Platonov, "The arithmetic theory of linear algebraic groups and number theory" Proc. Steklov Inst. Math. , 132 (1973) pp. 184–191 Trudy Mat. Inst. Steklov. , 132 (1973) pp. 162–168 Zbl 0305.20023
[9]	M. Kneser, "Starke Approximation in algebraischen Gruppen I" J. Reine Angew. Math. , 218 (1965) pp. 190–203 MR0184945 Zbl 0143.04701
[10]	M. Kneser, "Strong approximation" G.D. Mostow (ed.) A. Borel (ed.) , Proc. Symp. Pure Math. , 9 , Amer. Math. Soc. (1966) pp. 187–196 MR0213361 Zbl 0201.37904
[11]	M. Kneser, "Schwache Approximation in algebraischen Gruppen" , Colloq. Groupes Algébriques, Bruxelles , Gauthier-Villars (1962) pp. 41–52 Zbl 0171.29102
[12]	G. Harder, "Halbeinfache Gruppenschemata über Dedekindringen" Invent. Math. , 4 : 3 (1967) pp. 165–191
[13]	M. Eichler, "Allgemeine Kongruenzklasseneinteilungen der Ideale einfacher Algebren über algebraischen Zahlkörpern und ihre $L$-Reihen" J. Reine Angew. Math. , 179 (1938) pp. 227–251
[14]	H. Behr, "Zur starken Approximation in algebraischen Gruppen über globalen Körpern" J. Reine Angew. Math. , 229 (1968) pp. 107–116 MR0223371 Zbl 0184.24404
[15]	V.P. Platonov, "Reduced $K$-theory and approximation in algebraic groups" Proc. Steklov Inst. Math. , 142 : 3 (1979) pp. 213–224 Trudy Mat. Inst. Steklov. , 142 (1976) pp. 198–207
[16]	G. Prasad, "Strong approximation for semi-simple groups over function fields" Ann. of Math. (2) , 105 (1977) pp. 553–572 MR0444571 Zbl 0348.22006 Zbl 0344.22012

Comments

The Tamagawa number has been computed for every simple $G$. In particular, Weil's conjecture that $\tau(G) = 1$ if $G$ is simply connected has been shown to be true.