Quaternionic structure

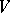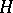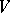A quaternionic structure on a real vector space is a module structure over the skew-field of quaternions , that is, a subalgebra of the algebra of endomorphisms of induced by two anti-commutative complex structures 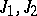on 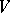(cf. Complex structure). The endomorphisms 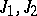are called standard generators of the quaternionic structure 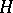, and the basis 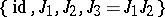of 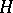defined by them is called the standard basis. A standard basis is defined up to automorphisms of 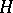. The algebra is isomorphic to the algebra of quaternions (cf. Quaternion). 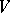An automorphism of the vector space is called an automorphism of the quaternionic structure if the transformation of the space of automorphisms induced by it preserves , that is, if 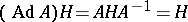. If, moreover, the identity automorphism is induced on 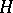, then 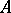is called a special automorphism of the quaternionic structure. The group of all special automorphisms of the quaternionic structure is isomorphic to the general linear group 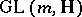over the skew-field , where 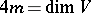. The group of all automorphisms of a quaternionic structure is isomorphic to the direct product with amalgamation of the subgroup 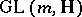and the group of unit quaternions .

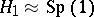A quaternionic structure on a differentiable manifold is a field of quaternionic structures on the tangent spaces, that is, a subbundle 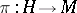of the bundle 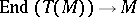of endomorphisms of tangent spaces whose fibres 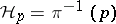are quaternionic structures on the tangent spaces for all . A pair of anti-commutative almost-complex structures 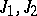on the manifold 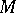is called a special quaternionic structure. It induces the quaternionic structure 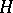, where

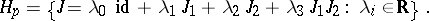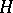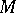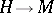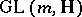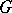A quaternionic structure on a manifold is induced by a special quaternionic structure if and only if the bundle is trivial. A quaternionic structure on a manifold can be regarded as a -structure, and a special quaternionic structure as a -structure in the sense of the theory of -structures (cf. -structure). Hence, in order that a quaternionic structure (or a special quaternionic structure) should exist on a manifold 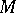, it is necessary and sufficient that the structure group of the tangent bundle reduces to the group 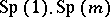(or 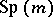). The first prolongation of a special quaternionic structure, regarded as a -structure, is an -structure (a field of frames), which determines a canonical linear connection associated with the special quaternionic structure. The vanishing of the curvature and torsion of this connection is a necessary and sufficient condition for the special quaternionic structure to be locally equivalent to the standard flat special quaternionic structure on the vector space .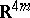

A quaternionic Riemannian manifold is the analogue of a Kähler manifold for quaternionic structures. It is defined as a Riemannian manifold 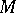of dimension 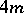whose holonomy group 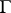is contained in the group 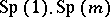. If 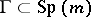, then the quaternionic Riemannian manifold is called a special or quaternionic Kähler manifold, and it has zero Ricci curvature. A quaternionic Riemannian manifold can be characterized as a Riemannian manifold 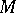in which there exists a quaternionic structure 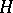that is invariant with respect to Levi-Civita parallel displacement. Similarly, a special quaternionic Riemannian manifold is a Riemannian manifold in which there exists a special quaternionic structure 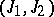that is invariant with respect to Levi-Civita parallel displacement: 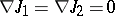, where is the operator of covariant differentiation of the Levi-Civita connection.

In a quaternionic Riemannian manifold there exists a canonical parallel 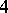-form that defines a number of operators in the ring 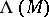of differential forms on 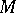that commute with the Laplace–Beltrami operator (exterior product operator, contraction operators). This enables one to construct an interesting theory of harmonic differential forms on quaternionic Riemannian manifolds [2] analogous to Hodge theory for Kähler manifolds, and to obtain estimates for the Betti numbers of the manifold 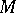(cf. Hodge structure; Betti number). Locally Euclidean spaces account for all the homogeneous special quaternionic Riemannian manifolds. As an example of a homogeneous quaternionic Riemannian manifold that is not special one may cite the quaternionic projective space and also other Wolf symmetric spaces which are in one-to-one correspondence with simple compact Lie groups without centre (cf. Symmetric space). These account for all compact homogeneous quaternionic Riemannian manifolds. A wide class of non-compact non-symmetric homogeneous quaternionic Riemannian manifolds can be constructed by means of modules over Clifford algebras (see [5]).

References

[1]	S.-S. Chern, "On a generalization of Kähler geometry" R.H. Fox (ed.) D.C. Spencer (ed.) A.W. Tucker (ed.) , Algebraic geometry and topology (Symp. in honor of S. Lefschetz) , Princeton Univ. Press (1957) pp. 103–121 MR0087172 Zbl 0078.14103
[2]	V.Y. Kraines, "Topology of quaternionic manifolds" Trans. Amer. Math. Soc. , 122 (1966) pp. 357–367 MR0192513 Zbl 0148.16101
[3]	K. Yano, M. Ako, "An affine connection in an almost quaternionic manifold" J. Differential Geom. , 8 : 3 (1973) pp. 341–347 MR355892
[4]	A.J. Sommese, "Quaternionic manifolds" Mat. Ann. , 212 (1975) pp. 191–214 MR0425827 Zbl 0299.53023
[5]	D.V. Alekseevskii, "Classification of quaternionic spaces with a transitive solvable group of motions" Math. USSR Izv. , 9 : 2 (1975) pp. 297–339 Izv. Akad. Nauk SSSR Ser. Mat. , 39 : 2 (1975) pp. 315–362 MR402649 Zbl 0324.53038
[6]	J.A. Wolf, "Complex homogeneous contact manifolds and quaternionic symmetric spaces" J. Math. Mech. , 14 : 6 (1965) pp. 1033–1047 MR0185554 Zbl 0141.38202
[7]	D.V. Aleksevskii, "Lie groups and homogeneous spaces" J. Soviet Math. , 4 : 5 (1975) pp. 483–539 Itogi Nauk. i Tekhn. Algebra. Topol. Geom. , 11 (1974) pp. 37–123