C-convexity
convexity in complex analysis
A domain or compact subset  in
in  is said to be
is said to be  -convex if for any complex line
-convex if for any complex line  the intersection
the intersection  is both connected and simply connected (meaning that its complement in the Riemann sphere
is both connected and simply connected (meaning that its complement in the Riemann sphere  is connected; cf. also Connected set; Simply-connected domain). The notion of
is connected; cf. also Connected set; Simply-connected domain). The notion of  -convexity is an intermediate one, in the sense that any geometrically convex set is necessarily
-convexity is an intermediate one, in the sense that any geometrically convex set is necessarily  -convex, whereas
-convex, whereas  -convexity implies holomorphic convexity (or pseudo-convexity; cf. also Pseudo-convex and pseudo-concave). In particular, a real subset
-convexity implies holomorphic convexity (or pseudo-convexity; cf. also Pseudo-convex and pseudo-concave). In particular, a real subset 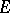 in
in 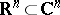 is
is  -convex if and only if it is convex in the ordinary, geometrical, sense. Open or compact
-convex if and only if it is convex in the ordinary, geometrical, sense. Open or compact 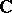 -convex subsets are in several respects the natural sets when it comes to studying properties of holomorphic functions that are invariant under affine (or projective) transformations. They often play a role analogous to that of convex sets in real analysis.
-convex subsets are in several respects the natural sets when it comes to studying properties of holomorphic functions that are invariant under affine (or projective) transformations. They often play a role analogous to that of convex sets in real analysis.
Topology of  -convex sets.
-convex sets.
Any 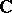 -convex domain is homeomorphically equivalent to the open unit ball
-convex domain is homeomorphically equivalent to the open unit ball  , and a compact
, and a compact 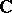 -convex set
-convex set 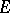 is also topologically simple in the sense that it has vanishing reduced cohomology:
is also topologically simple in the sense that it has vanishing reduced cohomology:
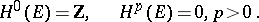 |
The operations of forming the closure and the interior are not well-adapted to  -convexity. There exist, e.g., compact
-convexity. There exist, e.g., compact  -convex sets with non-connected interior. Also, the intersection of two
-convex sets with non-connected interior. Also, the intersection of two  -convex sets is not necessarily
-convex sets is not necessarily  -convex.
-convex.
Projective invariance.
It is natural to consider a 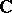 -convex set
-convex set 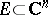 also as a subset of the complex projective space
also as a subset of the complex projective space  . Each non-trivial complex linear mapping
. Each non-trivial complex linear mapping 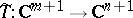 descends to a mapping
descends to a mapping  , where
, where  corresponds to the kernel of
corresponds to the kernel of 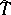 . The mapping
. The mapping  is then called a projective transformation, and
is then called a projective transformation, and  -convexity is invariant under any such transformation: If
-convexity is invariant under any such transformation: If 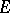 and
and 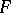 are
are  -convex subsets of
-convex subsets of 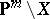 and
and  , respectively, then both
, respectively, then both 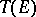 and
and 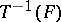 are also
are also  -convex.
-convex.
A  -convex set
-convex set 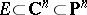 is said to be non-degenerate if it is not of the form
is said to be non-degenerate if it is not of the form  or
or 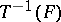 , where
, where 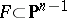 and
and 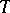 is a projective transformation. Examples of degenerate
is a projective transformation. Examples of degenerate 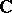 -convex sets are those that are contained in complex hyperplanes, or of the form
-convex sets are those that are contained in complex hyperplanes, or of the form  . It is an interesting fact that far from all one-dimensional
. It is an interesting fact that far from all one-dimensional  -convex sets can occur as the intersection of a multi-dimensional non-degenerate
-convex sets can occur as the intersection of a multi-dimensional non-degenerate 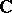 -convex set with a complex line.
-convex set with a complex line.
Dual complement and linear convexity.
If 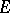 is any subset of
is any subset of  , then its dual complement
, then its dual complement 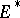 is, by definition, the collection of all complex hyperplanes that do not intersect
is, by definition, the collection of all complex hyperplanes that do not intersect  . When
. When  this is just the usual complement of a set in the Riemann sphere, and in higher dimensions
this is just the usual complement of a set in the Riemann sphere, and in higher dimensions  can be considered as a subset of the dual projective space
can be considered as a subset of the dual projective space  . If
. If  is an open (or compact)
is an open (or compact) 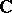 -convex set, then its dual complement
-convex set, then its dual complement 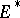 is a compact (respectively, open)
is a compact (respectively, open)  -convex set. Moreover, such a
-convex set. Moreover, such a  -convex set
-convex set 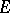 is also linearly convex in the sense that
is also linearly convex in the sense that 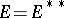 , i.e. the complement of
, i.e. the complement of 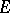 is a union of complex hyperplanes. There are, however, many linearly convex sets that are not
is a union of complex hyperplanes. There are, however, many linearly convex sets that are not 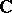 -convex. For instance, any Cartesian product of subsets of
-convex. For instance, any Cartesian product of subsets of  is linearly convex, but it is
is linearly convex, but it is  -convex only if each factor is convex in the usual sense. A connected component of a linearly convex set
-convex only if each factor is convex in the usual sense. A connected component of a linearly convex set 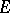 is not necessarily linearly convex. In fact, a compact or open set
is not necessarily linearly convex. In fact, a compact or open set 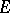 which is equal to one or several connected components of its linearly convex hull
which is equal to one or several connected components of its linearly convex hull 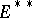 is said to be weakly linearly convex. For an open set
is said to be weakly linearly convex. For an open set 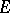 , weak linear convexity amounts to the condition that through any boundary point
, weak linear convexity amounts to the condition that through any boundary point  there should pass a complex hyperplane not intersecting
there should pass a complex hyperplane not intersecting  , and this does not in general imply linear convexity of
, and this does not in general imply linear convexity of  . Any weakly linearly convex open set
. Any weakly linearly convex open set 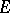 in
in 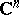 is pseudo-convex.
is pseudo-convex.
Boundary properties.
If a weakly linearly open set in 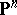 ,
,  , has a boundary of class
, has a boundary of class 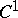 , then it is automatically
, then it is automatically  -convex. In particular, for bounded domains in
-convex. In particular, for bounded domains in 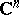 with
with  boundary, the notions of
boundary, the notions of 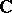 -convexity, linear convexity and weak linear convexity all coincide.
-convexity, linear convexity and weak linear convexity all coincide.
When the smoothness assumption is strengthened, so that 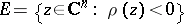 is given by a defining function
is given by a defining function  of class
of class  , then one considers the quadratic form
, then one considers the quadratic form
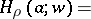 |
 |
where  ,
, 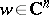 ,
,  , and
, and 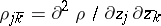 . This quadratic form is called the Hessian of
. This quadratic form is called the Hessian of 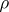 at
at  , whereas its Hermitian part
, whereas its Hermitian part
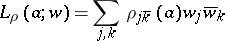 |
is called the Levi form (cf. also Hessian of a function). A smoothly bounded domain 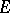 is convex if and only if the Hessian of its defining function is positive semi-definite when restricted to the real tangent plane at any
is convex if and only if the Hessian of its defining function is positive semi-definite when restricted to the real tangent plane at any 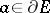 . Similarly, a domain
. Similarly, a domain  is pseudo-convex precisely if the restriction of the Levi form to the complex tangent plane is positive semi-definite. The notion of
is pseudo-convex precisely if the restriction of the Levi form to the complex tangent plane is positive semi-definite. The notion of 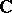 -convexity lies in between: A domain
-convexity lies in between: A domain  with boundary of class
with boundary of class 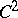 is
is 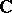 -convex if and only if for any
-convex if and only if for any 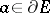 the Hessian is positive semi-definite on the complex tangent plane at
the Hessian is positive semi-definite on the complex tangent plane at  .
.
A complex hyperplane is said to be a tangent plane to an arbitrary open set  if it intersects the boundary
if it intersects the boundary 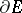 but not
but not 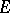 itself. When
itself. When  , a connected open set
, a connected open set 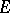 is
is 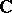 -convex if and only if, for any
-convex if and only if, for any 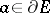 , the set of complex tangent planes to
, the set of complex tangent planes to 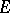 at
at  is a non-empty connected subset of the dual complement
is a non-empty connected subset of the dual complement 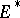 .
.
Fantappiè transform.
If 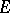 is an open (or compact) subset of
is an open (or compact) subset of 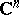 , one denotes by
, one denotes by 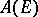 the vector space of holomorphic functions on
the vector space of holomorphic functions on  , endowed with the projective (respectively, inductive) limit topology. An element
, endowed with the projective (respectively, inductive) limit topology. An element 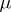 of the dual space
of the dual space 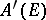 is called an analytic functional on
is called an analytic functional on  . If
. If  contains the origin, then any hyperplane not intersecting
contains the origin, then any hyperplane not intersecting  is of the form
is of the form  , and the Fantappiè transform
, and the Fantappiè transform 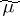 is defined to be the element of
is defined to be the element of 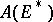 given by
given by
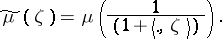 |
Now, if 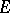 is
is 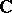 -convex, then the mapping
-convex, then the mapping  provides a topological isomorphism between the spaces
provides a topological isomorphism between the spaces 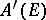 and
and 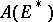 . For
. For  there is a converse: If
there is a converse: If  is holomorphically convex and if
is holomorphically convex and if 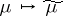 is bijective, then
is bijective, then 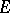 must in fact be
must in fact be 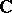 -convex. Cf. Duality in complex analysis.
-convex. Cf. Duality in complex analysis.
The surjectivity of the Fantappiè transform is closely related to integral representation formulas for holomorphic functions (cf. also Integral representations in multi-dimensional complex analysis). Let 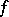 be holomorphic in a neighbourhood of a
be holomorphic in a neighbourhood of a  -convex compact set
-convex compact set 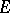 , and let
, and let  be a smoothly bounded small open neighbourhood of
be a smoothly bounded small open neighbourhood of 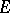 . By the Michael selection theorem one may choose, for every
. By the Michael selection theorem one may choose, for every 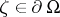 , a hyperplane
, a hyperplane  depending in a smooth way on
depending in a smooth way on 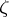 . Letting
. Letting  also denote the differential form
also denote the differential form 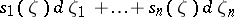 , one then has the Cauchy–Fantappiè formula
, one then has the Cauchy–Fantappiè formula
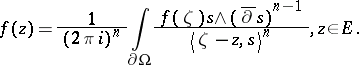 |
Here, the integral kernel is homogeneous in  , so one can replace
, so one can replace  by the new section
by the new section 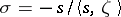 and then obtain
and then obtain
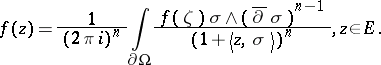 |
This integral formula has the following discrete analogue: Any function  , holomorphic in a neighbourhood of a
, holomorphic in a neighbourhood of a 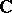 -convex compact set
-convex compact set  , has a decomposition into partial fractions
, has a decomposition into partial fractions
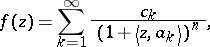 |
where all 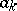 are contained in some compact subset of
are contained in some compact subset of 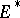 , and
, and 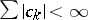 . Hence the series converges uniformly in a neighbourhood of
. Hence the series converges uniformly in a neighbourhood of 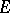 .
.
If 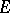 is only assumed to be weakly linearly convex, then the partial fraction representation becomes
is only assumed to be weakly linearly convex, then the partial fraction representation becomes
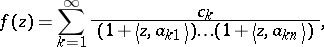 |
again with 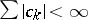 and uniform convergence in a neighbourhood of
and uniform convergence in a neighbourhood of 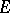 . Conversely, any holomorphically convex compact
. Conversely, any holomorphically convex compact 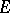 admitting such representations must necessarily be weakly linearly convex.
admitting such representations must necessarily be weakly linearly convex.
Existence of primitive functions.
For any vector  , let
, let 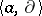 denote the first-order differential operator
denote the first-order differential operator
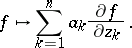 |
This directional derivative can, of course, be viewed as a mapping  , where
, where 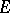 is any domain in
is any domain in 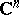 . If
. If 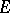 is
is  -convex, then this mapping is surjective for any
-convex, then this mapping is surjective for any  , and conversely, if
, and conversely, if 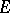 is bounded and the mapping
is bounded and the mapping 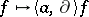 is surjective for each
is surjective for each  , then
, then 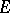 must be
must be  -convex. More generally, consider any linear differential operator
-convex. More generally, consider any linear differential operator 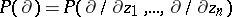 with constant coefficients, not all equal to zero, and regard it as a mapping
with constant coefficients, not all equal to zero, and regard it as a mapping 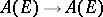 . Then, if
. Then, if 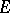 is
is 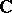 -convex, this mapping is surjective.
-convex, this mapping is surjective.
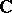 -convexity in other contexts.
-convexity in other contexts.
Sets that are 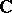 -convex occur naturally in several other connections, such as in complex polynomial approximation (in particular, in so-called Kergin interpolation), invariant metrics and pluri-potential theory, for instance in the work of L. Lempert. The fact that the dual complement of a convex set in
-convex occur naturally in several other connections, such as in complex polynomial approximation (in particular, in so-called Kergin interpolation), invariant metrics and pluri-potential theory, for instance in the work of L. Lempert. The fact that the dual complement of a convex set in 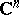 is not necessarily convex, but only
is not necessarily convex, but only  -convex, is also a motivation for considering
-convex, is also a motivation for considering 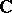 -convex sets.
-convex sets.
Terminology.
There is a certain confusion in the literature as to the terminology connected with 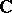 -convexity and the related convexity notions. The first ones to study weak linear convexity were H. Behnke and E. Peschl [a4], who used the term Planarkonvexität. Later, A. Martineau [a8] introduced the term convexité linéelle for what is here called linear convexity. At about the same time, L. Aizenberg [a2] and his school in Krasnoyarsk took up the Russian expression lineinaya vypuklost', which in English translations became "linear convexity" , to denote what is here called weak linear convexity. Some authors prefer to use the English adjective "lineal" rather than linear in this context, stressing the fact that the (original) French term is "linéel" and not "linéaire" . Until the 1990s, strong linear convexity was the term used for
-convexity and the related convexity notions. The first ones to study weak linear convexity were H. Behnke and E. Peschl [a4], who used the term Planarkonvexität. Later, A. Martineau [a8] introduced the term convexité linéelle for what is here called linear convexity. At about the same time, L. Aizenberg [a2] and his school in Krasnoyarsk took up the Russian expression lineinaya vypuklost', which in English translations became "linear convexity" , to denote what is here called weak linear convexity. Some authors prefer to use the English adjective "lineal" rather than linear in this context, stressing the fact that the (original) French term is "linéel" and not "linéaire" . Until the 1990s, strong linear convexity was the term used for 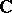 -convexity, whereas projective complement and conjugate set were used as synonyms for the dual complement
-convexity, whereas projective complement and conjugate set were used as synonyms for the dual complement 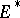 . In the above, the terminology adopted by L.V. Hörmander [a6] is followed.
. In the above, the terminology adopted by L.V. Hörmander [a6] is followed.
References
| [a1] | L. Aizenberg, "Decomposition of holomorphic functions of several complex variables into partial fractions" Sib. Math. J. , 8 (1967) pp. 859–872 |
| [a2] | L. Aizenberg, "Linear convexity in 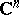 and the separation of singularities of holomorphic functions" Bull. Acad. Polon. Sci. Ser. Math. Astr. Phys. , 15 (1967) pp. 487–495 (In Russian) (French summary) and the separation of singularities of holomorphic functions" Bull. Acad. Polon. Sci. Ser. Math. Astr. Phys. , 15 (1967) pp. 487–495 (In Russian) (French summary) |
| [a3] | M. Andersson, M. Passare, R. Sigurdsson, "Complex convexity and analytic functionals I" Science Inst. Univ. Iceland Preprint , June (1995) |
| [a4] | H. Behnke, E. Peschl, "Zur Theorie der Funktionen mehrerer komplexer Veränderlichen. Konvexität in bezug auf analytische Ebenen im kleinen und grossen" Math. Ann. , 111 (1935) pp. 158–177 MR1512986 Zbl 0011.16903 Zbl 61.0373.01 |
| [a5] | S.G. Gindikin, G.M. Henkin, "Integral geometry for 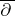 -cohomologies in -cohomologies in 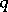 -linearly concave domains in -linearly concave domains in  " Funct. Anal. Appl. , 12 (1978) pp. 247–261 " Funct. Anal. Appl. , 12 (1978) pp. 247–261 |
| [a6] | L. Hörmander, "Notions of convexity" , Progr. Math. , 127 , Birkhäuser (1994) MR1301332 Zbl 0835.32001 |
| [a7] | C.O. Kiselman, "A differential inequality characterizing weak lineal convexity" Math. Ann. , 311 (1998) pp. 1–10 MR1624326 Zbl 0911.32031 |
| [a8] | A. Martineau, "Sur la topologie des espaces de fonctions holomorphes" Math. Ann. , 163 (1966) pp. 62–88 MR0190697 Zbl 0138.38101 |
| [a9] | A. Martineau, "Sur la notion d'ensemble fortement linéellement convexe" An. Acad. Brasil. Ci. , 40 (1968) pp. 427–435 MR0282292 |
| [a10] | S.V. Znamenskii, "A geometric criterion for strong linear convexity" Funct. Anal. Appl. , 13 (1979) pp. 224–225 MR545375 |
| [a11] | S.V. Znamenskii, L.N. Znamenskaya, "Spiral connectedness of the sections and projections of 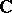 -convex sets" Math. Notes , 59 (1996) pp. 253–260 -convex sets" Math. Notes , 59 (1996) pp. 253–260 |
C-convexity. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=C-convexity&oldid=24394