Norm map

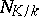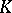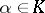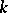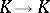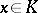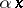The mapping of a field into a field , where is a finite extension of (cf. Extension of a field), that sends an element to the element that is the determinant of the matrix of the -linear mapping that takes to . The element 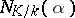is called the norm of the element .

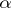One has 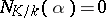if and only if 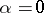. For any ,

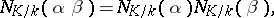that is, 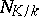induces a homomorphism of the multiplicative groups 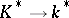, which is also called the norm map. For any ,

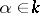The group is called the norm subgroup of , or the group of norms (from 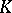into ). If is the characteristic polynomial of 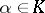relative to 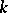, then

Suppose that is separable (cf. Separable extension). Then for any ,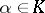

where the 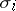are all the isomorphisms of into the algebraic closure of fixing the elements of $k$.

The norm map is transitive. If and 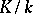are finite extensions, then

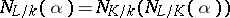for any .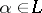

References

[1]	S. Lang, "Algebra" , Addison-Wesley (1984)
[2]	Z.I. Borevich, I.R. Shafarevich, "Number theory" , Acad. Press (1966) (Translated from Russian) (German translation: Birkhäuser, 1966)