Lebedev-Skal'skaya transform

Skal'skaya–Lebedev transform

The integral transform

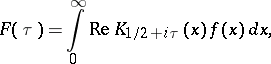(a1)

where

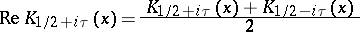and 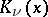is the Macdonald function. This transformation was introduced by N.N. Lebedev and I.P. Skal'skaya and investigated in connection with possible applications to certain problems in mathematical physics. It is also called the -transform.

The -transform was initiated by them as well:

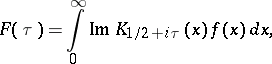(a2)

where

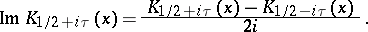If 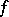is an integrable function on with respect to the weight 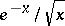, i.e. , then the Lebedev–Skal'skaya transforms (a1), (a2) exist and represent bounded continuous functions on the positive half-axis which tend to zero at infinity (an analogue of the Riemann–Lebesgue lemma, cf. also Fourier series).

Let 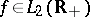. Then the Lebedev–Skal'skaya transforms (a1), (a2) converge in the mean-square sense to functions belonging to the space and isomorphically map these two spaces onto each other. Moreover, the Parseval equality holds (see [a5])

as well as the inversion formulas

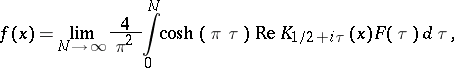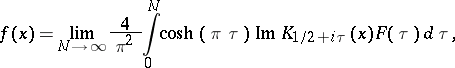for the two transforms, respectively, where the integrals are understood in the mean-square sense.

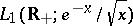If two functions , are from the space , then 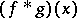defines a convolution (cf. also Convolution of functions), for instance for the Lebedev–Skalskaya transform (a1),

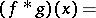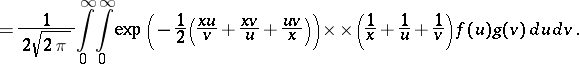The convolution belongs to the same space 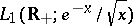and satisfies the norm estimate

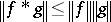in this space. The result of the action of the Lebedev–Skal'skaya transform (a1) on this convolution gives the product 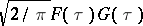, where 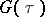is the Lebedev–Skal'skaya transform of the function .

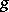If, moreover, 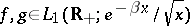, , 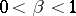then the following integral representation of the convolution holds:

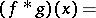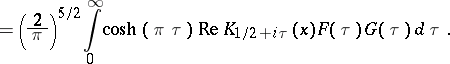References

[a1]	N.N. Lebedev, N.P. Skalskaya, "Some integral transforms related to the Kontorovich–Lebedev transform" Probl. Math. Phys. St. Petersburg (1976) pp. 68–79 (In Russian)
[a2]	Yu.M. Rappoport, "Integral equations and Parseval equalities for the modified Kontorovich–Lebedev transforms" Diff. Uravn. , 17 (1981) pp. 1697–1699 (In Russian)
[a3]	S.B. Yakubovich, "On the new properties of the Kontorovich–Lebedev like integral transforms" Rev. Tec. Ing. Univ. Zulia , 18 : 3 (1995) pp. 291–299
[a4]	S.B. Yakubovich, Yu.F. Luchko, "The hypergeometric approach to integral transforms and convolutions" , Kluwer Acad. Publ. (1994)
[a5]	S.B. Yakubovich, "Index transforms" , World Sci. (1996)