Gell-Mann-Dashen algebra
An infinite-dimensional Lie algebra occurring in quantum field theory. Let  be a finite-dimensional Lie algebra and
be a finite-dimensional Lie algebra and 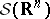 the space of Schwartz test functions (cf. Generalized functions, space of). The Lie algebra
the space of Schwartz test functions (cf. Generalized functions, space of). The Lie algebra  is defined by
is defined by
 |
and can be interpreted as the Lie algebra of the group of gauge transformations (cf. Gauge transformation) [a1]. Representations of  are called current algebras in quantum field theory. Let
are called current algebras in quantum field theory. Let 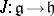 be a homomorphism of Lie algebras and let
be a homomorphism of Lie algebras and let 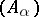 be a basis of
be a basis of 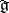 with structure constants
with structure constants  . The mapping
. The mapping 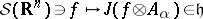 defines an
defines an 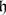 -valued distribution
-valued distribution 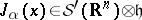 and it is true that
and it is true that
 |
Passing to the Fourier image one sets 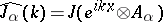 for
for  ; then
; then
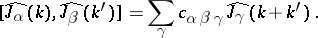 |
R. Dashen and M. Gell-Mann (1966) studied and applied the latter commutation relations in the particular case when 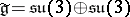 , [a2].
, [a2].
General references for current algebras are [a3], [a4].
References
| [a1] | R. Hermann, "Lie algebras and quantum mechanics" , Benjamin (1970) |
| [a2] | R. Dashen, M. Gell-Mann, "Representation of local current algebra at infinite momentum" Phys. Rev. Lett. , 17 (1966) pp. 340–343 |
| [a3] | S.L. Adler, R. Dashen, "Current algebras" , Benjamin (1968) |
| [a4] | B. Renner, "Current algebras and their applications" , Pergamon (1968) |
Gell-Mann-Dashen algebra. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Gell-Mann-Dashen_algebra&oldid=22503