Renormalization group analysis

The theoretical physicist aims to elaborate theories at the microscopic scale, from which observed phenomena can be explained. Renormalization group analysis allows one to determine effective theories at each length scale, from microscopic to macroscopic, by averaging over "degrees of freedom" of the previous scale. For instance, given a system defined on a lattice of spacing (e.g., is a lattice of spacing in ) and a Lagrangian, or a Hamiltonian, involving an interaction between sites, a new Lagrangian is obtained on a lattice of spacing by summing over possible values of the initial variables at each site of , for given values of variables on the new lattice, etc. In some theories, e.g. few-body Newtonian mechanics, "degrees of freedom" at very different scales "decouple" ; as a consequence, particles (or planets in the solar system) can be approximated by point-like objects in large-scale analysis. This is no longer true in quantum field theory in particle physics, nor in the related study of phase transitions and critical phenomena in classical statistical physics, domains in which renormalization group analysis has been mainly developed. The renormalization group, which is actually a semi-group, is the set of transformations, in an infinite-dimensional space of Lagrangians, that give the effective Lagrangian of each scale from those of shorter scales. In cases studied, effective Lagrangians tend to fixed points; moreover, there will exist "universality classes" such that the effective Lagrangians will all tend to a common fixed point or to a set of fixed points of finite dimension. The dominant long-distance physics will then depend only on a finite number of parameters.

-models.

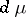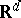In field theory, a model is defined by a probability measure on the space of fields . Fields are functions or distributions defined on , or on a lattice in . They have real-valued components, . Correlation functions are the moments of , e.g., for . In applications, is either the usual space or Euclidean space-time. (In the latter case, real-time physics follows by analytic continuation in time variables: .)

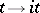Given a Lagrangian 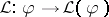, 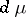has the heuristic ill-defined form , or 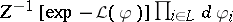. In the 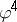-model on 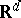, for ,

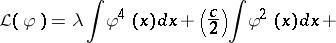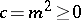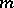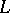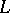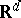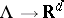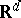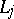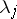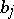with integrals replaced by discrete sums (and derivatives by differences) on a lattice. At , , it describes a free theory of mass . To define the model on a lattice one may first restrict to a finite volume of and then consider the limit as . On one may initially consider in lattices with spacings and parameters , , , and consider the continuous limit as 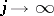. 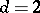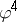As explained below, this will allow one, for and , to define the -model and will yield an effective Lagrangian on a lattice of spacing in 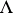. The limit as is treated in turn.

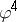-models in a finite volume 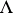of .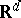

For any given 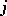, renormalization group analysis yields Lagrangians 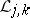, , 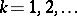on lattices with spacings 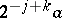, including terms in , , 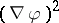with coefficients 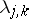, , , plus (already at ) infinitely many other terms in 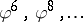. However, the latter will be "irrelevant" . Given , , 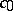, 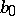it is then expected at or that will be well defined in the limit as 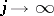, with 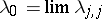, , 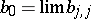if , , are suitably chosen; and remain close to and , while 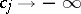. The results are confirmed by a rigorous analysis in which a truncated version of effective Lagrangians (involving first terms only) is used.

The theory is asymptotically free at short distances (the weight of the interaction, in , tends to as ).

There is no result at , the physical dimension in particle physics; a conjecture is that only more refined "gauge" theories can then exist.

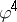-models on a lattice.

(The limit as 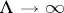.) Effective theories are obtained on lattices with spacings , 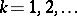. Two-point correlations decrease exponentially as 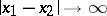in "massive" cases (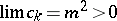) and as inverse powers of 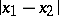in "critical" mass-less cases ().

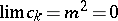i) There exists a such that the theory is critical. For 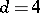, as 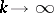: asymptotic freedom at large distances follows with a trivial (Gaussian) fixed point and a dominant long-distance behaviour in as in the free 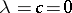theory.

For 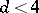and large, a non-trivial fixed point is obtained as 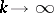, by means of 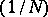expansions: dominant behaviour, at 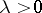, in 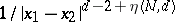, with a critical exponent 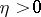independent of (the "anomalous dimensionanomalous dimension" ). These results can be proved under suitable conditions.

On the other hand, a heuristic analysis for any 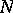can be made using -expansions. One considers a space dimension 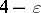. For 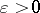small, a non-trivial fixed point close to the Gaussian fixed point for 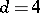is obtained. Results are then extended to (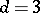) and (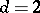). The critical indices obtained at 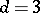or 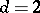are close to the experimental ones in related situations, or to those obtained from numerical calculations, or also, at 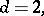to exact results on the related Ising model.

ii) For one obtains a massive Gaussian fixed point.

iii) For 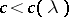there is a discrete symmetry breaking at 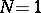: in dependence on, e.g., boundary conditions on 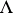, different non-trivial fixed points, which are mixtures of two pure "phases" are obtained in the limit as 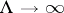(symmetry then relates different solutions).

For 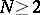, there is for 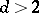a continuous symmetry breaking: an infinite number of non-trivial fixed points linked by a "Goldstone bosonGoldstone boson" .

Physical theories.

Applications in statistical physics (cf. also Statistical physics, mathematical problems in) related to the previous models include phase transitions: liquid-vapour (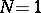), superfluid helium (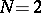), ferromagnetic systems (), and statistical properties of long polymers ( "N= 0" ). Among other applications of renormalization group analysis is the BCS theory of superconductivity. In particle physics, the theory of strong interactions (QCD) makes sense as an asymptotically free theory at short distances; the situation is different for theories of strong and electroweak interactions, which might be viewed as effective theories arising from a more fundamental theory at "ultrashort" distances.

Applications of renormalization group ideas have been made in many other domains; of particular mathematical interest are the recent studies of partial differential equations using this method.

For general references on renormalization group analysis, see [a1], [a2]; see [a3] for rigorous results.

References

[a1]	K.G. Wilson, J. Kogut, "The renormalization group and the -expansion" Phys. Rep. , 12 (1974) pp. 75–200
[a2]	J. Zinn-Justin, "Quantum field theory and critical phenomena" , Oxford Univ. Press (1996) (Edition: Third)
[a3]	J. Magnen, D. Iagolnitzer (ed.) , XI-th Internat. Congress Math. Physics , Internat. Press , Boston (1995) pp. 121–141