Trigonometric system
From Encyclopedia of Mathematics
One of the most important orthogonal systems of functions (cf. Orthogonal system). The functions of the trigonometric system,
 |
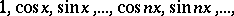are orthogonal on any interval of the form 
, while the functions
 |
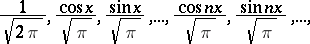are orthonormal on this interval. The trigonometric system is complete and closed in the space 
for 
, and also in the space 
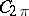of continuous 
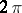-periodic functions. This system forms a basis in 
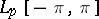for 
. Series in the trigonometric system are studied in the theory of trigonometric series.
Alongside the trigonometric system, wide use is made of the complex trigonometric system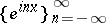 
. The functions of these systems are related to one another by the Euler formulas.
References
| [1] | N.K. [N.K. Bari] Bary, "A treatise on trigonometric series" , Pergamon (1964) (Translated from Russian) |
| [2] | A. Zygmund, "Trigonometric series" , 1–2 , Cambridge Univ. Press (1988) |
How to Cite This Entry:
Trigonometric system. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Trigonometric_system&oldid=19218
Trigonometric system. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Trigonometric_system&oldid=19218
This article was adapted from an original article by B.I. Golubov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article