Darbo fixed-point theorem
The notion of "measure of non-compactness" was first introduced by C. Kuratowski [a1]. For any bounded set  in a metric space its measure of non-compactness, denoted by
in a metric space its measure of non-compactness, denoted by  , is defined to be the infimum of the positive numbers
, is defined to be the infimum of the positive numbers  such that
such that  can be covered by a finite number of sets of diameter less than or equal to
can be covered by a finite number of sets of diameter less than or equal to  .
.
Another measure of non-compactness is the ball measure  , or Hausdorff measure, which is defined as the infimum of the positive numbers
, or Hausdorff measure, which is defined as the infimum of the positive numbers  such that
such that  can be covered by a finite number of balls of radii smaller than
can be covered by a finite number of balls of radii smaller than  . See also Hausdorff measure.
. See also Hausdorff measure.
Roughly speaking, a measure of non-compactness is some function defined on the family of all non-empty bounded subsets of a given metric space such that it is equal to zero on the whole family of relatively compact sets.
G. Darbo used a measure of non-compactness to investigate operators whose properties can be characterized as being intermediate between those of contraction and compact mappings (cf. also Compact mapping; Compact operator; Contraction). He was the first to use the index  in the theory of fixed points [a2]. Darbo's fixed-point theorem is a generalization of the well-known Schauder fixed-point theorem (cf. also Schauder theorem). It states that if
in the theory of fixed points [a2]. Darbo's fixed-point theorem is a generalization of the well-known Schauder fixed-point theorem (cf. also Schauder theorem). It states that if 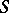 is a non-empty bounded closed convex subset of a Banach space
is a non-empty bounded closed convex subset of a Banach space  and
and  is a continuous mapping such that for any set
is a continuous mapping such that for any set 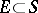 ,
,
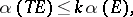 | (a1) |
where 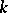 is a constant,
is a constant,  , then
, then  has a fixed point. This theorem is true for the measure
has a fixed point. This theorem is true for the measure 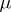 also.
also.
Note that every completely-continuous mapping (or compact mapping; cf. also Completely-continuous operator) satisfies (a1) with  , while all Lipschitz mappings with constant
, while all Lipschitz mappings with constant  (cf. Lipschitz condition) also satisfy (a1). Further, mappings that are not completely continuous but satisfy the condition (a1) are of the form
(cf. Lipschitz condition) also satisfy (a1). Further, mappings that are not completely continuous but satisfy the condition (a1) are of the form 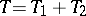 , where
, where  is completely continuous and
is completely continuous and 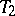 satisfies the Lipschitz condition with constant
satisfies the Lipschitz condition with constant 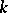 . The significance of this type of mapping is due to the fact that compactness of either the domain or the range is not required.
. The significance of this type of mapping is due to the fact that compactness of either the domain or the range is not required.
Methods for determining the value of 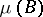 for a given set
for a given set 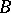 in a Banach space are given in [a3].
in a Banach space are given in [a3].
Darbo's fixed-point theorem is useful in establishing the existence of solutions of various classes of differential equations, especially for implicit differential equations, integral equations and integro-differential equations, see [a3]. It is also used to study the controllability problem for dynamical systems represented by implicit differential equations [a4].
References
| [a1] | C. Kuratowski, "Sur les espaces complets" Fundam. Math. , 15 (1930) pp. 301–309 |
| [a2] | G. Darbo, "Punti uniti in transformazioni a condominio non compacto" Rend. Sem. Mat. Univ. Padova , 24 (1955) pp. 84–92 |
| [a3] | J. Banas, K. Goebel, "Measure of noncompactness in Banach spaces" , M. Dekker (1980) |
| [a4] | K. Balachandran, J.P. Dauer, "Controllability of nonlinear systems via fixed point theorems" J. Optim. Th. Appl. , 53 (1987) pp. 345–352 |
Darbo fixed-point theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Darbo_fixed-point_theorem&oldid=19165