Thinness of a set

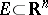at a point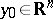

A local criterion for the fact that 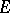is a polar set. A non-empty set 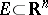is said to be thin at the point 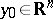in two cases:

1) 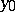is not a limit point of 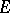; that is, 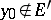, where 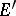is the derived set of ;

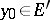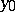2) and there exists a superharmonic function in a neighbourhood of (see Superharmonic function) such that

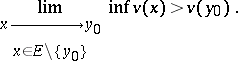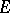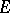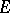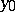The set is polar if and only if it is thin at each of its points. For an arbitrary set the subset of those points at which is thin is polar. Any non-empty subset of a set which is thin at the point is thin at . The union of a finite number of sets thin at the point 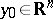is a set thin at .

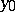A segment in the plane 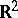is not a thin set at any of its points. If 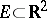is a thin set at a point , then there exist arbitrarily small discs with centre 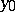and not intersecting 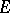. A polar set 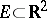is completely discontinuous. However, the Cantor set on the -axis (which is of measure zero) is not thin at any of its points. At the same time, for example, in 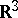the set of points

which has a spine at the point 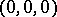, where

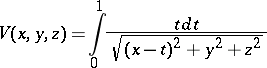is the Newton potential with density 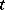on the segment 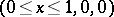, is a thin set at the spine 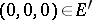(Lebesgue's example).

References

[1]	M. Brélot, "Eléments de la théorie classique du potentiel" , Sorbonne Univ. Centre Doc. Univ. , Paris (1969)
[2]	N.S. Landkof, "Foundations of modern potential theory" , Springer (1972) (Translated from Russian)

Comments

Two further interesting properties of thinness are: 1) 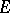is thin at if and only if is not a limit point of with respect to the fine topology; and 2) a boundary point of an open set , bounded if 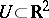, is regular for the Dirichlet problem if and only if the complement of 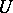is not thin at .

The concept of thinness, and its use to define a fine topology, is fundamental in any potential theory. For example, in probabilistic potential theory associated to a strong Markov process, a Borel set 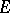is thin at if and only if, starting from , the process almost surely will not hit 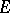even once. But, in general, a set thin at each of its points is not polar; a countable union of such sets is called a semi-polar set, a kind of exceptional set (related to the Dirichlet problem) which can be considerably bigger than a polar set when the potential theory lacks symmetry (for example, for the heat equation potential theory). Roughly speaking, a set 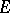is polar (respectively semi-polar) in probabilistic potential theory if the process almost surely never meets 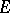(respectively, only meets 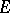at most a countable number of times). See also Potential theory, abstract.

References

[a1]	C. Constantinescu, A. Cornea, "Potential theory on harmonic spaces" , Springer (1972)