Developable surface

torse

A ruled surface of zero Gaussian curvature. At all the points on one generator a developable surface has the same tangent plane. The distribution parameter of a developable surface is zero. If the generators of a developable surface are parallel to the same straight line, the surface is a cylinder. If the generators all pass through one point, the surface is a cone. In the remaining cases the developable surface is formed by the tangents to a certain space curve — the cuspidal edge (or edge of regression) of the developable surface. In this case the curvature lines are given by the straight line generators and their orthogonal trajectories.

A developable surface is the envelope of a one-parameter family of planes (for example, a rectifying surface) and therefore is locally obtained by isometrically deforming a piece of a plane.

Comments

Let 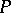be a non-flat point (cf. Flat point) on a (not necessarily ruled) surface 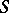of zero Gaussian curvature. Then locally around 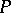, the surface 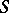is developable. Cf. Ruled surface for the notions of generators and distribution parameters (of a ruled surface).

References

[a1]	C.C. Hsiung, "A first course in differential geometry" , Wiley (1981) pp. Chapt. 3, Sect. 4
[a2]	W. Blaschke, K. Leichtweiss, "Elementare Differentialgeometrie" , Springer (1973)