Lie ternary system
From Encyclopedia of Mathematics
A vector space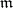 
with a trilinear composition
 |
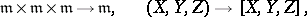satisfying the following conditions:
 |
 |
 |
 |
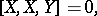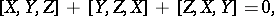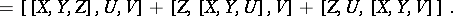If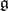 
is a Lie algebra and 
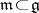is a subspace such that 
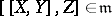for any 
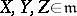, then the operation
 |
converts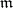 
into a Lie ternary system. Conversely, every Lie ternary system can be obtained in this way from some Lie algebra.
The category of finite-dimensional Lie ternary systems over the field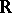 
is equivalent to the category of simply-connected symmetric homogeneous spaces (see Symmetric space).
References
| [1] | S. Helgason, "Differential geometry, Lie groups, and symmetric spaces" , Acad. Press (1978) |
| [2] | O. Loos, "Symmetric spaces" , 1 , Benjamin (1969) |
How to Cite This Entry:
Lie ternary system. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lie_ternary_system&oldid=18380
Lie ternary system. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lie_ternary_system&oldid=18380
This article was adapted from an original article by A.S. Fedenko (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article