Residue of an analytic function

of one complex variable at a finite isolated singular point of unique character

The coefficient of 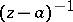in the Laurent expansion of the function 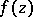(cf. Laurent series) in a neighbourhood of , or the integral

where 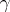is a circle of sufficiently small radius with centre at , which is equal to it. The residue is denoted by .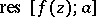

The theory of residues is based on the Cauchy integral theorem. The residue theorem is fundamental in this theory. Let be a single-valued analytic function everywhere in a simply-connected domain 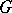, except for isolated singular points; then the integral of 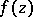over any simple closed rectifiable curve lying in and not passing through the singular points of 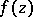can be computed by the formula

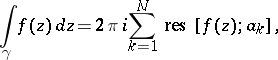where 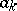, , 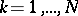are the singular points of 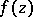inside .

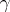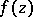The residue of a function at the point at infinity , for a function which is single-valued and analytic in a neighbourhood of that point, is defined by the formula

where 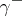is a circle of sufficiently large radius, oriented clockwise, while is the coefficient of 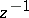in the Laurent expansion of 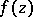in a neighbourhood of the point at infinity. The residue theorem implies the theorem on the total sum of residues: If 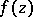is a single-valued analytic function in the extended complex plane, except for a finite number of singular points, then the sum of all residues of 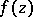, including the residue at the point at infinity, is zero.

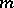Thus, the computation of integrals of analytic functions along closed curves (contour integrals) is reduced to the computation of residues, which is particularly simple in the case of finite poles. Let be a pole of order of the function 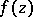(cf. Pole (of a function)); then

If (a simple pole), the formula becomes

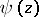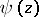if , where and are regular in a neighbourhood of , and if is a simple zero for , then

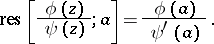The application of the residue theorem to the logarithmic derivative yields the important theorem on logarithmic residues: If a function 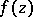is meromorphic in a simply-connected domain , while the simple closed curve 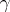lies in and does not pass through zeros or poles of 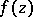, then

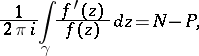where 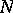is the number of zeros and is the number of poles of 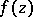inside 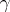counted with multiplicities. The expression on the left-hand side of the formula is called the logarithmic residue of the function with respect to the curve 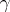(see also Argument, principle of the).

Residues are employed in computing certain integrals of real-valued functions, such as

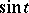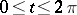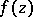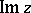where is a rational function of , which is continuous if , and is a continuous function if , where is the imaginary part of , and is analytic if except for a finite number of singular points. By substituting 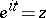, is reduced to the contour integral

i.e. to the computation of the residues;

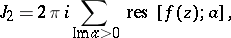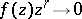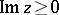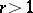if as , , ; and

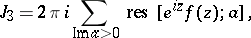if 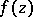satisfies the conditions of the Jordan lemma.

Residues have found numerous important applications in problems of analytic continuation, decomposition of meromorphic functions into partial fractions, summation of power series, asymptotic estimation, and many other problems of theoretical and applied analysis [1]–[4].

The theory of residues in one variable was mostly developed by A.L. Cauchy in 1825–1829. A number of results concerning the generalizations of the theory were obtained by Ch. Hermite (a theorem on the sum of the residues of doubly-periodic functions), P. Laurent, Yu.V. Sokhotskii, E. Lindelöf, and others.

Residues of analytic differentials rather than residues of analytic functions are studied on Riemann surfaces [5] (cf. also Differential on a Riemann surface). The residue of an analytic differential 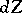in a neighbourhood of (one of) its isolated singular points is defined as the coefficient of in the Laurent expansion of the function 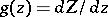, where is a uniformizing parameter (cf. Uniformization) in a neighbourhood of this point. The integral of along any closed curve on the Riemann surface can be expressed in terms of the residues of the differential and its cyclic periods (the integrals of along canonical cuts, cf. Canonical sections). The theorem on the total sum of residues is applicable to Riemann surfaces: The sum of all residues of a meromorphic differential on a compact Riemann surface is zero.

The theory of residues of analytic functions of several complex variables.

See [8]–[10], [12], [13]. This theory is based on the integral theorems of Stokes and Cauchy–Poincaré, which make it possible to replace the integral of a closed form along one cycle by an integral of this form along another cycle which is homologous to the former. The foundations of the theory of residues of functions of several variables were laid by H. Poincaré [6], who was the first (1887) to generalize Cauchy's integral theorem and the concept of a residue to functions of two complex variables; he showed, in particular, that the integral of a rational function of two complex variables along a two-dimensional cycle which does not pass through the singularities of the integrand can be reduced to the periods of Abelian integrals (cf. Abelian integral), and employed double residues as the basis of a two-dimensional analogue of Lagrange series.

J. Leray [7] (see also [4], [8]) developed the general theory of residues on a complex-analytic manifold . Leray's residue theory describes, in particular, a method of computing integrals along certain cycles on 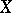of closed exterior differential forms with singularities on analytic submanifolds. He introduced the concept of a residue form, which generalizes the concept of a residue of an analytic function of a single variable; the residue formula thus obtained makes it possible to reduce the computation of the integral of a form 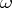with a first-order polar singularity on a complex-analytic submanifold 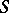along a given cycle in to the computation of an integral of the residue form along a cycle on 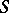of one dimension lower. In calculating integrals of closed forms with arbitrary singularities on 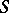, the important concepts are those of a residue class (cf. Residue form) and the Leray theorem, according to which any closed form 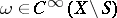has a corresponding cohomologous form 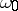with a first-order polar singularity on . For a form 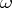with a singularity on several submanifolds 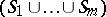one uses the composite residue form

the residue class

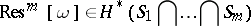and the residue formula

where 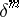is the composite Leray coboundary operator associated to the Leray coboundary operator 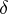and 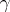is a cycle in .

There exists another approach to the theory of residues of functions of several complex variables — the method of distinguishing a homology basis, based on an idea of E. Martinelli and involving the use of Alexander duality [8]. Let 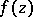, , 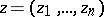be a holomorphic function in a domain 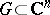, and let 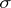be an -dimensional cycle in 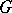. If is a basis of the -dimensional homology space of the domain 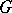and

is the expansion of 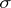with respect to this basis, a generalization of the residue theorem has the form

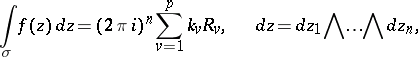where

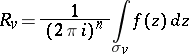is an -dimensional analogue of the residue and is called the residue of the function with respect to the basic cycle . As distinct from the case of one variable, it is very difficult to find both a homology basis 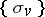and the coefficients 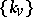. In several cases (for example, when , where is a polynomial) these problems may be solved with the aid of Alexander–Pontryagin duality. The coefficients are found as the linking coefficients of the cycle with the cycles on the set (compactified in a certain manner) which are dual to the cycles 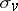. The residues 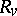can in some cases be found as the respective coefficients of the Laurent expansion of the function .

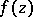Multi-dimensional analogues of logarithmic residues [4], [8]–[9] express the number of common zeros (counted with multiplicities) of a system of holomorphic functions in a domain 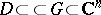by means of the integrals

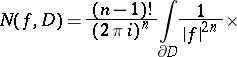where 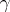is some cycle in 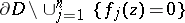. Residues of functions of several variables have found use in the study of Feynman integrals, in combinatorial analysis [11] and in the theory of implicit functions [8].

References

[1]	A.I. Markushevich, "Theory of functions of a complex variable" , 1 , Chelsea (1977) (Translated from Russian)
[2]	M.A. Evgrafov, "Analytic functions" , Saunders , Philadelphia (1966) (Translated from Russian)
[3]	I.I. [I.I. Privalov] Priwalow, "Einführung in die Funktionentheorie" , 1–3 , Teubner (1958–1959) (Translated from Russian)
[4]	B.V. Shabat, "Introduction of complex analysis" , 1–2 , Moscow (1985) (In Russian)
[5]	G. Springer, "Introduction to Riemann surfaces" , Addison-Wesley (1957) pp. Chapt.10
[6]	H. Poincaré, "Sur les résidues des intégrales doubles" Acta Math. , 9 (1887) pp. 321–380
[7]	J. Leray, "Le calcule différentiel et intégral sur une variété analytique complexe (Problème de Cauchy, III)" Bull. Soc. Math. France , 87 (1959) pp. 81–180
[8]	L.A. Aizenberg, A.P. Yuzhakov, "Integral representations and residues in multidimensional complex analysis" , Transl. Math. Monogr. , 58 , Amer. Math. Soc. (1983) (Translated from Russian)
[9]	A.K. Tsikh, "Multidimensional residues and its applications" , Amer. Math. Soc. (Forthcoming) (Translated from Russian)
[10]	P.A. Griffiths, "On the periods of certain rational integrals I" Ann. of Math. (2) , 90 : 3 (1969) pp. 460–495
[11]	G.P. Egorichev, "Integral representation and the computation of combinatorial sums" , Amer. Math. Soc. (1984) (Translated from Russian)
[12]	P.A. Griffiths, J.E. Harris, "Principles of algebraic geometry" , Wiley (Interscience) (1978)
[13]	W.R. Coleff, M.F. Herrera, "Les courants residuals associés à une forme meromorphe" , Lect. notes in math. , 633 , Springer (1978)

Comments

See also the comments and references to Residue form.

References

[a1]	D.S. Mitrinović, J.D. Kečkić, "The Cauchy method of residues: theory and applications" , Reidel (1984)