Unimodular element

unimodular vector

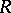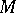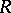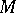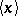Let be a ring with unit and a right module over . An element in is called unimodular if and the submodule generated by has a complement in , i.e. there is a submodule 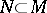such that , , so that .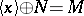

An element of a free module 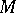that is part of a basis of 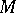is unimodular. An element 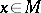is unimodular if and only if there is a homomorphism of modules 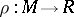such that 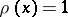. A row (or column) of a unimodular matrix over 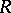is unimodular. The question when the converse is true is important in algebraic -theory. Cf. also Stable rank.

References

[a1]	A.J. Hahn, O.T. O'Meara, "The classical groups and 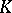-theory" , Springer (1989) pp. 9, §141ff