Omega-squared distribution

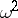-distribution

The probability distribution of the random variable

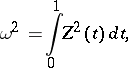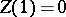where is a conditional Wiener process (conditioned on ). The characteristic function of the "omega-squared" distribution is expressed by the formula

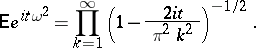In mathematical statistics, the "omega-squared" distribution is often found in the following circumstances. Let be independent random variables, uniformly distributed on 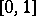, according to which an empirical distribution function 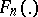is constructed. In this case, the process

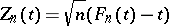converges weakly to a conditional Wiener process, from which it follows that

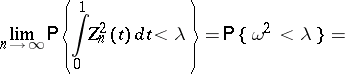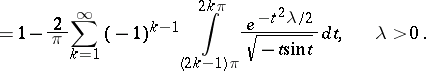See also Cramér–von Mises test.

References

[1]	N.V. Smirnov, "On the 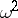-distribution" Mat. Sb. , 2 (1937) pp. 973–993 (In Russian)
[2]	T.W. Anderson, D.A. Darling, "Asymptotic theory of certain "goodness of fit" criteria based on stochastic processes" Ann. Math. Stat. , 23 (1952) pp. 193–212

Comments

The "conditional Wiener process" 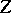is usually referred to in the Western literature as tied-down Brownian motion, pinned Brownian motion or as the Brownian bridge.

The pioneering paper is [a1].

References

[a1]	D.A. Darling, "The Cramér–Smirnov test in the parametric case" Ann. Math. Stat. , 26 (1955) pp. 1–20
[a2]	J. Durbin, "Distribution theory for tests based on the sample distribution function" , SIAM (1973)