Jordan criterion
From Encyclopedia of Mathematics
for the convergence of Fourier series
If a 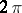 -periodic function
-periodic function 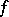 has bounded variation on the interval
has bounded variation on the interval  , then its Fourier series converges at every point
, then its Fourier series converges at every point 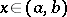 to
to 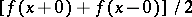 ; if, in addition,
; if, in addition, 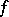 is continuous on
is continuous on  , then its Fourier series converges to it uniformly on every interval
, then its Fourier series converges to it uniformly on every interval 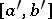 strictly inside
strictly inside  . The criterion was established by C. Jordan [1]; it generalizes the Dirichlet theorem on the convergence of Fourier series of piecewise-monotone functions.
. The criterion was established by C. Jordan [1]; it generalizes the Dirichlet theorem on the convergence of Fourier series of piecewise-monotone functions.
References
| [1] | C. Jordan, "Sur la série de Fourier" C.R. Acad. Sci. Paris , 92 (1881) pp. 228–230 |
| [2] | N.K. [N.K. Bari] Bary, "A treatise on trigonometric series" , Pergamon (1964) (Translated from Russian) |
Comments
The Jordan criterion is also called the Dirichlet–Jordan test, cf. [a1].
References
| [a1] | A. Zygmund, "Trigonometric series" , 1–2 , Cambridge Univ. Press (1988) |
How to Cite This Entry:
Jordan criterion. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Jordan_criterion&oldid=17285
Jordan criterion. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Jordan_criterion&oldid=17285
This article was adapted from an original article by B.I. Golubov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article