Unitary transformation

unitary mapping

A linear transformation of a unitary space preserving the inner product of vectors, i.e. such that for any vectors and of one has the equality

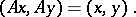A unitary transformation preserves, in particular, the length of a vector. Conversely, if a linear transformation of a unitary space preserves the lengths of all vectors, then it is unitary. The eigenvalues of a unitary transformation have modulus 1; the eigenspaces corresponding to different eigenvalues are orthogonal.

A linear transformation of a finite-dimensional unitary space is unitary if and only if it satisfies any of the following conditions:

1) in any orthonormal basis the transformation corresponds to a unitary matrix;

2) maps any orthonormal basis to an orthonormal basis;

3) in 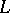there exists an orthonormal basis of eigenvectors of 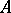, and, moreover, 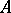has in this basis a diagonal matrix with diagonal entries of modulus 1.

The unitary transformations of a given unitary space form a group under multiplication of transformations (called the unitary group).

References

[a1]	W.H. Greub, "Linear algebra" , Springer (1975) pp. 338ff