Weak topology
From Encyclopedia of Mathematics
The locally convex topology on a vector space 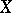 generated by the family of semi-norms (cf. Semi-norm)
generated by the family of semi-norms (cf. Semi-norm)  , where
, where 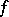 ranges over some subset
ranges over some subset  of the (algebraic) adjoint space
of the (algebraic) adjoint space 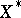 .
.
References
| [1] | L.A. Lyusternik, V.I. Sobolev, "A short course of functional analysis" , Moscow (1982) (In Russian) |
| [2] | H.H. Schaefer, "Topological vector spaces" , Springer (1971) |
Comments
The weak topology as introduced above is often denoted by 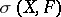 . It is a Hausdorff topology if and only if
. It is a Hausdorff topology if and only if 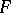 separates the points of
separates the points of 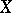 .
.
See also Strong topology.
References
| [a1] | H. Jarchow, "Locally convex spaces" , Teubner (1981) (Translated from German) |
How to Cite This Entry:
Weak topology. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Weak_topology&oldid=17244
Weak topology. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Weak_topology&oldid=17244
This article was adapted from an original article by M.I. Voitsekhovskii (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article