Topological dynamics
The branch of the theory of dynamical systems in which one studies topological dynamical systems (cf. Dynamical system; Topological dynamical system). The basic situation is the case where the phase space is a metric compactum, and time runs through  ,
,  or
or  (this is assumed throughout).
(this is assumed throughout).
The origins of topological dynamics (1920–1930) were connected with the fact that a series of concepts concerning the limiting behaviour of a trajectory (for example, the limit set and the centre of a topological dynamical system) and the "repetitiveness" of motion can usefully be discussed in the general context of topological dynamics, although these concepts themselves arose in the study of more concrete objects — differentiable dynamical systems. Various "repetitiveness" properties are (in increasing order of generality): periodicity, almost-periodicity (in the sense of Bohr), recurrence (in the sense of Birkhoff, cf. Minimal set, 2)), Poisson stability, non-wanderingness (cf. Wandering point), and chain recurrence. As time increases, any trajectory approaches the set formed by "repetitive" trajectories; from this point of view the special attention given to the latter is completely justified.
In the 1960's the study of minimal sets and their extensions led to a significant development of topological dynamics as an independent discipline. (This was primarily connected with distal dynamical systems, cf. Distal dynamical system.) However, one must bear in mind that the study of the limiting behaviour of trajectories does not reduce simply to the study of minimal sets.
Up to the end of the 1960's, topological dynamics dealt mainly with the questions listed above (cf. also the following paragraph, and [1], [6], [11], – in Dynamical system). However, in many cases it turned out to be necessary to take account of "non-repetitive" trajectories as well. (Thus, there is often interest in separatrices.) In particular, they can describe waves of a certain special kind, irrespective of whether or not they are chain recurrent. The gradient dynamical systems (cf. Gradient dynamical system) corresponding to functions with non-degenerate critical points are used in the study of manifolds; however, in this case the set of "repetitive" trajectories has a trivial structure. Therefore, later the behaviour of trajectories outside the set of "repetitive" motions was also studied, as were compact invariant sets which are isolated, or locally maximal: in some neighbourhood  of the set there is no larger invariant set. Among the trajectories in such a set, there may be "non-repetitive" ones. For such sets one can introduce a certain analogue of the Morse index (no longer a number, but a more complicated object) and establish a relationship that generalizes the Morse inequalities (just as in the corresponding result for a Morse–Smale system). One discusses also the behaviour of such sets under continuous changes in the system. (Since one is not interested in these questions in the future of a trajectory which leaves
of the set there is no larger invariant set. Among the trajectories in such a set, there may be "non-repetitive" ones. For such sets one can introduce a certain analogue of the Morse index (no longer a number, but a more complicated object) and establish a relationship that generalizes the Morse inequalities (just as in the corresponding result for a Morse–Smale system). One discusses also the behaviour of such sets under continuous changes in the system. (Since one is not interested in these questions in the future of a trajectory which leaves  , it is possible, and sometimes even necessary, to consider "local dynamical systems" , in which the "motion" of a point need not be defined for all values of time. The precise formulation is somewhat unwieldy. Cf. [1], [10].)
, it is possible, and sometimes even necessary, to consider "local dynamical systems" , in which the "motion" of a point need not be defined for all values of time. The precise formulation is somewhat unwieldy. Cf. [1], [10].)
Related to topological dynamics are questions about invariant measures (cf. Invariant measure); topological entropy; asymptotic cycles (cf. [2], [3]); or the question to which class in the sense of descriptive set theory some subset or other of the phase space, naturally determined by its properties, belongs (cf. , [5]). In topological dynamics, as generally in the theory of dynamical systems, one asks questions about those properties of dynamical systems which are in a certain sense "generic" (cf. [9], [10] in General position, and [3] in Chain recurrence, and also [6], [7]). (In fact, topological dynamics is only concerned with "genericity" in the  -topology, and for differentiable dynamical systems,
-topology, and for differentiable dynamical systems,  -topology,
-topology,  , is appropriate on a correspondingly smaller set of systems.) In the framework of topological dynamics one can discuss the relationship between Lyapunov stability and various related notions.
, is appropriate on a correspondingly smaller set of systems.) In the framework of topological dynamics one can discuss the relationship between Lyapunov stability and various related notions.
The application of topological dynamics to concrete dynamical systems or classes of them is largely connected with the use of concepts and results of topological dynamics in the theory of differentiable dynamical systems (partly also in ergodic theory). In such applications of topological dynamics one often has to consider systems in which the phase space is not a manifold. (This happens when topological dynamics is applied to the restriction of a dynamical system to an invariant set which is not a manifold, or in the use of symbolic dynamics, or when both these situations are combined.) From the middle of the 1960's, and especially in the 1980's, a lot of work was done on cascades obtained by iterating a continuous mapping  of an interval or circle. A lot of this does not depend on
of an interval or circle. A lot of this does not depend on 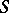 being smooth, and is not connected with invariant measures, so relates to "pure" topological dynamics.
being smooth, and is not connected with invariant measures, so relates to "pure" topological dynamics.
Topological dynamics has been used (cf. [8], [11]) for a unified approach to a series of number-theoretical results (although for the deepest of them one has to refer to ergodic theory). Finally, although the definition of a topological flow recalls many of the properties of an autonomous system of ordinary differential equations, topological dynamics can sometimes also be applied indirectly in the study of non-autonomous systems (here the system is considered along with a continuum of other systems obtained from it by a certain limiting process, and a construction arises analogous to that of a skew product 2), cf. [9]).
References
| [1] | C. Conley, "Isolated invariant sets and the Morse index" , Amer. Math. Soc. (1978) |
| [2] | S. Schwartzman, "Asymptotic cycles" Ann. of Math. , 66 (1957) pp. 270–284 |
| [3] | F. Rhodes, "Asymptotic cycles for continuous curves on geodesic spaces" J. London Math. Soc. , 6 (1973) pp. 247–255 |
| [4a] | O.M. Sharkovskii, "On cycles and the structure of a continuous mapping" Ukr. Mat. Zh. , 17 : 3 (1965) pp. 104–111 (In Russian) |
| [4b] | O.M. Sharkovskii, "A classification of fixed points" Ukr. Mat. Zh. , 17 : 5 (1965) pp. 80–95 (In Russian) |
| [4c] | O.M. Sharkovskii, "The behaviour of an iteration in a neighbourhood of a fixed point" Ukr. Mat. Zh. , 18 : 2 (1966) pp. 60–83 (In Russian) |
| [4d] | O.M. Sharkovskii, "Attractive sets not containing limit cycles" Ukr. Math. J. , 20 : 1 (1968) pp. 131–134 Ukr. Mat. Zh. , 20 : 1 (1968) pp. 136–142 |
| [5] | O.M. Sharkovskii, "The convergence set of one-dimensional iterations" Dop. Akad. Nauk. UkrSSR : 7 (1966) pp. 866–870 ((in Ukrainian. English abstract)) |
| [6] | V.A. Dobrynskii, "Genericity of dynamical systems with stable propagation" , Dynamical systems and stability questions in the solution of differential equations , Kiev (1973) pp. 43–53 (In Russian) |
| [7] | J. Palis, C. Pugh, M. Shub, D. Sullivan, "Genericity theorems in topological dynamics" A. Manning (ed.) , Dynamical systems (Warwick, 1974) , Lect. notes in math. , 468 , Springer (1975) pp. 241–250 |
| [8] | H. Furstenberg, "Poincaré recurrence and number theory" Bull. Amer. Math. Soc. , 5 : 3 (1981) pp. 211–234 |
| [9] | V.M. Millionshchikov, "Les systèmes linéaires d'équations différentielles ordinaires" , Proc. Internat. Congress Mathematicians (Nice, 1970) , 1 , Gauthier-Villars (1971) pp. 915–920 |
| [10] | D. Salamon, "Connected simple systems and the Conley index of isolated invariant sets" Trans. Amer. Math. Soc. , 291 : 1 (1985) pp. 1–41 |
| [11] | H. Furstenberg, "Recurrence in ergodic theory and combinatorial number theory" , Princeton Univ. Press (1981) |
Comments
The study of minimal sets and their extensions is carried out also for the case that the phase space is not metric (but still compact) and "time" runs through an arbitrary topological group; see also [a1]. For cascades obtained by iterating a continuous mapping of the interval or circle, see e.g. [a2]–[a4], [a6].
For non-autonomous systems, see also [a5] and Chapt. IV in
of Dynamical system.
References
| [a1] | J. Auslander, "Minimal flows and their extensions" , North-Holland (1988) |
| [a2] | R. Bowen, J. Franks, "The periodic points of maps of the disk and the interval" Topology , 15 (1976) pp. 337–342 |
| [a3] | M. Martens, S. van Strien, W. de Melo, "Julia—Fatou—Sullivan theory for real one-dimensional dynamics" Acta Math. (To appear) |
| [a4] | B. Thurston, "On iterated maps of the interval" J.C. Alexander (ed.) , Dynamical Systems (Proc. Maryland, 1986–7) , Lect. notes in math. , 1342 , Springer (1988) pp. 465–563 |
| [a5] | G.R. Sell, "Topological dynamics and ordinary differential equations" , v. Nostrand (1971) |
| [a6] | A.N. Sharkovskii, "Coexistence of cycles of continuous maps of a line into itself" Ukrain. Mat. Zh. , 16 (1964) pp. 61–71 (In Russian) |
| [a7] | G.A. Hecklund, "Topological dynamics" , Amer. Math. Soc. (1955) |
| [a8] | S. Smale, "The mathematics of time" , Springer (1980) |
Topological dynamics. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Topological_dynamics&oldid=17008