Representation of a compact group(2)
A homomorphism of a compact group into the group of continuous linear automorphisms of a (complex) Banach space that is continuous with respect to the strong operator topology.
Let  be a compact group, let
be a compact group, let  be a Banach space and let
be a Banach space and let  be a representation. If
be a representation. If  is a Hilbert space and
is a Hilbert space and  is a unitary operator for every
is a unitary operator for every  , then
, then  is called a unitary representation. There always is an equivalent norm in
is called a unitary representation. There always is an equivalent norm in  for which
for which  is unitary.
is unitary.
Every irreducible unitary representation (cf. Irreducible representation) of a compact group 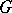 is finite-dimensional. Let
is finite-dimensional. Let  be the family of all possible pairwise inequivalent irreducible unitary representations of the group
be the family of all possible pairwise inequivalent irreducible unitary representations of the group 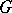 . Every unitary representation
. Every unitary representation 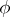 of
of  is an orthogonal sum of unique representations
is an orthogonal sum of unique representations  ,
, 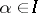 , such that
, such that 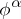 is an orthogonal sum, possibly zero, of a set of representations equivalent to
is an orthogonal sum, possibly zero, of a set of representations equivalent to  .
.
If  is finite, then the family
is finite, then the family  is also finite and contains as many elements as there are distinct conjugacy classes in
is also finite and contains as many elements as there are distinct conjugacy classes in  (moreover,
(moreover,  ). The problem of studying these representations (computing their characters, finding explicit realizations, etc.) is the subject of an extensive theory (cf. Finite group, representation of a).
). The problem of studying these representations (computing their characters, finding explicit realizations, etc.) is the subject of an extensive theory (cf. Finite group, representation of a).
If  is a connected, simply-connected, compact Lie group and
is a connected, simply-connected, compact Lie group and  is its complexification (cf. Complexification of a Lie group), then the description of the family
is its complexification (cf. Complexification of a Lie group), then the description of the family  for
for  amounts (by restricting the representations to
amounts (by restricting the representations to 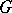 ) to the description of the family of all irreducible pairwise inequivalent finite-dimensional rational representations of the reductive algebraic group
) to the description of the family of all irreducible pairwise inequivalent finite-dimensional rational representations of the reductive algebraic group  . The latter family, in turn, allows of a complete description by considering highest weights (cf. Representation with a highest weight vector).
. The latter family, in turn, allows of a complete description by considering highest weights (cf. Representation with a highest weight vector).
In modern number theory and algebraic geometry one considers  -adic representations of compact totally-disconnected groups (cf. [5], [6]).
-adic representations of compact totally-disconnected groups (cf. [5], [6]).
References
| [1] | L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1958) (Translated from Russian) |
| [2] | M.A. Naimark, "Theory of group representations" , Springer (1982) (Translated from Russian) |
| [3] | D.P. Zhelobenko, "Compact Lie groups and their representations" , Amer. Math. Soc. (1973) (Translated from Russian) |
| [4] | S. Lang, " " , Addison-Wesley (1975) " , Addison-Wesley (1975) |
| [5] | I.M. Gel'fand, M.I. Graev, I.I. Pyatetskii-Shapiro, "Generalized functions" , 6. Representation theory and automorphic functions , Saunders (1969) (Translated from Russian) |
| [6] | J.-P. Serre, "Cohomologie Galoisienne" , Springer (1964) |
| [7] | C. Chevalley, "Theory of Lie groups" , 1 , Princeton Univ. Press (1946) |
Comments
References
| [a1] | N. Bourbaki, "Groupes et algèbres de Lie" , Eléments de mathématiques , Masson (1982) pp. Chapt. 9. Groupes de Lie réels compacts |
| [a2] | Th. Bröcker, T. Tom Dieck, "Representations of compact Lie groups" , Springer (1985) |
| [a3] | E. Hewitt, K.A. Ross, "Abstract harmonic analysis" , II , Springer (1970) |
| [a4] | A. Wawrzyńczyk, "Group representations and special functions" , Reidel & PWN (1984) |
Representation of a compact group(2). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Representation_of_a_compact_group(2)&oldid=16204