Riemann differential equation
A linear homogeneous ordinary differential equation of the second order in the complex plane with three given regular singular points (cf. Regular singular point)  ,
,  and
and  having characteristic exponents
having characteristic exponents  ,
,  ,
,  at these points. The general form of such an equation was first given by E. Papperitz, because of which it is also known as a Papperitz equation. Solutions of a Riemann differential equation are written in the form of the so-called Riemann
at these points. The general form of such an equation was first given by E. Papperitz, because of which it is also known as a Papperitz equation. Solutions of a Riemann differential equation are written in the form of the so-called Riemann  -function
-function
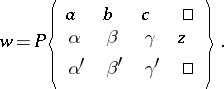 |
Riemann differential equations belong to the class of Fuchsian equations (cf. Fuchsian equation) with three singular points. A particular case of Riemann differential equations is the hypergeometric equation (the singular points are 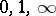 ); therefore, a Riemann differential equation itself is sometimes known as a generalized hypergeometric equation. A Riemann differential equation can be reduced to a Pochhammer equation, and its solution can thus be written in the form of an integral over a special contour in the complex plane.
); therefore, a Riemann differential equation itself is sometimes known as a generalized hypergeometric equation. A Riemann differential equation can be reduced to a Pochhammer equation, and its solution can thus be written in the form of an integral over a special contour in the complex plane.
For references see Papperitz equation.
Riemann differential equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Riemann_differential_equation&oldid=15928