Multilinear mapping

-linear mapping, multilinear operator

A mapping 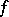of the direct product 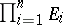of unitary modules 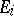(cf. Unitary module) over a commutative associative ring with a unit into a certain 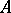-module 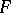which is linear in each argument, i.e. which satisfies the condition

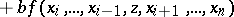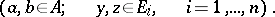In the case () one speaks of a bilinear mapping (respectively, a trilinear mapping). Each multilinear mapping

defines a unique linear mapping 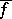of the tensor product 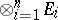into 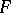such that

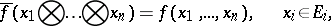where the correspondence is a bijection of the set of multilinear mappings 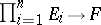into the set of all linear mappings 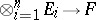. The multilinear mappings naturally form an -module.

On the 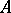-module 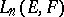of all -linear mappings 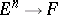there acts the symmetric group :

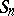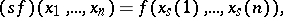where , , 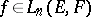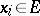. A multilinear mapping is called symmetric if 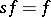for all , and skew-symmetric if 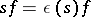, where in accordance with the sign of the permutation . A multilinear mapping is called sign-varying (or alternating) if 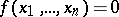when for some 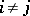. Any alternating multilinear mapping is skew-symmetric, while if in the equation 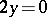has the unique solution 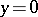the converse also holds. The symmetric multilinear mappings form a submodule in 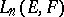that is naturally isomorphic to the module of linear mappings 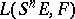, where 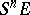is the -th symmetric power of (see Symmetric algebra). The alternating multilinear mappings form a submodule that is naturally isomorphic to 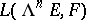, where is the -th exterior power of the module 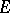(see Exterior algebra). The multilinear mapping 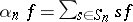is called the symmetrized multilinear mapping defined by 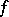, while the multilinear mapping 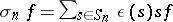is called the skew-symmetrized mapping defined by 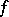. Symmetrized (skew-symmetrized) multilinear mappings are symmetric (respectively, alternating), and if in 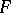the equation 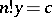has a unique solution for each 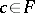, then the converse is true. A sufficient condition for any alternating multilinear mapping to be a skew-symmetrization is that the module is free (cf. Free module). For references see Multilinear form.