Approximation in the mean

Approximation of a given function 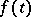, integrable on an interval 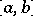, by a function 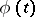, where the quantity

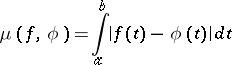is taken as the measure of approximation.

The more general case, when

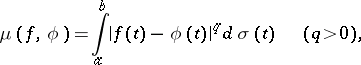where is a non-decreasing function different from a constant on 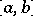, is called mean-power approximation (with exponent 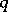) with respect to the distribution . If is absolutely continuous and 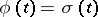, then one obtains mean-power approximation with weight 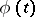, and if is a step function with jumps 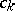at points 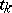in 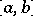, one has weighted mean-power approximation with respect to the system of points 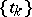with measure of approximation

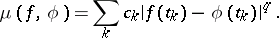These concepts are extended in a natural way to the case of functions of several variables.

References

[1]	V.L. Goncharov, "The theory of interpolation and approximation of functions" , Moscow (1954) (In Russian)
[2]	S.M. Nikol'skii, "Approximation of functions of several variables and imbedding theorems" , Springer (1975) (Translated from Russian)
[3]	J.R. Rice, "The approximation of functions" , 1. Linear theory , Addison-Wesley (1964)

References

[a1]	A.F. Timan, "Theory of approximation of functions of a real variable" , Pergamon (1963) (Translated from Russian)
[a2]	T.J. Rivlin, "An introduction to the approximation of functions" , Dover, reprint (1981)
[a3]	E.W. Cheney, "Introduction to approximation theory" , Chelsea, reprint (1982) pp. 203ff