Lie algebra of an analytic group
Lie algebra of a Lie group 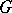 defined over a field
defined over a field 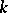 that is complete with respect to a non-trivial absolute value
that is complete with respect to a non-trivial absolute value
The Lie algebra 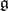 of
of 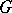 regarded as local Lie group (cf. Lie group, local). Thus, as a vector space
regarded as local Lie group (cf. Lie group, local). Thus, as a vector space  is identified with the tangent space to
is identified with the tangent space to 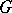 at the point
at the point  . The multiplication operation
. The multiplication operation 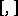 in the Lie algebra
in the Lie algebra  can be defined in any of the following equivalent ways.
can be defined in any of the following equivalent ways.
1) Let ad be the differential of the adjoint representation of the group 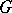 (cf. Adjoint representation of a Lie group). Then
(cf. Adjoint representation of a Lie group). Then 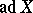 , for any vector
, for any vector 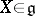 , is a linear transformation of the space
, is a linear transformation of the space 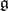 and
and  for any
for any 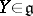 .
.
2) Let 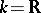 , let
, let  be two tangent vectors to
be two tangent vectors to 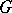 at
at  and let
and let 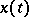 and
and  be smooth curves in
be smooth curves in 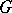 for which
for which 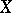 and
and 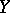 are tangent vectors for
are tangent vectors for 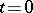 . Then
. Then 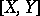 is the tangent vector for
is the tangent vector for 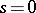 to the curve
to the curve 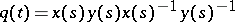 , where
, where  and
and 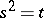 .
.
3) Let  be the associative
be the associative  -algebra of generalized functions on
-algebra of generalized functions on 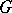 with support at
with support at  and with multiplication defined by the convolution
and with multiplication defined by the convolution  . The space
. The space 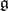 is identified with the set of primitive elements (cf. Hopf algebra) of the bi-algebra
is identified with the set of primitive elements (cf. Hopf algebra) of the bi-algebra 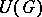 , and for any
, and for any 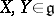 the vector
the vector 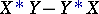 also lies in
also lies in  . Then
. Then  .
.
4) Let 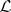 be the vector space of all vector fields on
be the vector space of all vector fields on  that are invariant with respect to left translation by elements of
that are invariant with respect to left translation by elements of  . The correspondence between the vector field and its value at the point
. The correspondence between the vector field and its value at the point 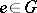 is an isomorphism of the vector spaces
is an isomorphism of the vector spaces 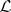 and
and 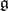 . On the other hand, to any vector field
. On the other hand, to any vector field 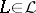 corresponds a left-invariant derivation of the
corresponds a left-invariant derivation of the  -algebra
-algebra 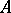 of analytic functions on
of analytic functions on 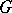 by means of the formula
by means of the formula 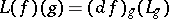 for any
for any 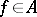 ,
, 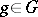 , and this correspondence is an isomorphism of the space
, and this correspondence is an isomorphism of the space 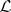 to the vector space
to the vector space  of all left-invariant derivations of
of all left-invariant derivations of  . For any
. For any 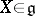 , let
, let 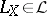 denote the left-invariant vector field for which
denote the left-invariant vector field for which 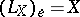 . If
. If 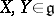 , then the product
, then the product  can be defined as the vector of
can be defined as the vector of 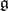 for which the field
for which the field 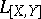 specifies the derivation
specifies the derivation 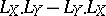 of the algebra
of the algebra 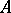 .
.
Example. Let 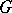 be the analytic group of all non-singular matrices of order
be the analytic group of all non-singular matrices of order  with coefficients in
with coefficients in 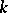 . Then the tangent space
. Then the tangent space 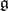 to
to  at the identity is identified with the space of all matrices of order
at the identity is identified with the space of all matrices of order  with coefficients in
with coefficients in 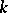 , and a Lie algebra structure on
, and a Lie algebra structure on 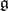 is defined by the formula
is defined by the formula 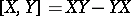 .
.
The correspondence between an analytic group and its Lie algebra has important functorial properties and significantly reduces the study of analytic groups to the study of their Lie algebras. Namely, let 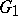 and
and 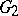 be analytic groups with Lie algebras
be analytic groups with Lie algebras 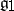 and
and 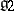 and let
and let  be an analytic homomorphism. Then
be an analytic homomorphism. Then  is a homomorphism of Lie algebras. The Lie algebra of the analytic group
is a homomorphism of Lie algebras. The Lie algebra of the analytic group 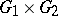 is isomorphic to
is isomorphic to 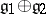 . If
. If  is the Lie algebra of an analytic group
is the Lie algebra of an analytic group 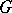 ,
,  is a Lie subgroup of
is a Lie subgroup of 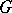 (see Lie group) and
(see Lie group) and  is the Lie algebra of the analytic group
is the Lie algebra of the analytic group  , then
, then 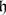 is a subalgebra of
is a subalgebra of 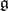 , while if
, while if 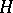 is normal, then
is normal, then 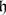 is an ideal of
is an ideal of 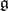 . Suppose that the characteristic of
. Suppose that the characteristic of 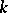 is zero. The Lie algebra of an intersection of Lie subgroups coincides with the intersection of their Lie algebras. The Lie algebra of the kernel of a homomorphism
is zero. The Lie algebra of an intersection of Lie subgroups coincides with the intersection of their Lie algebras. The Lie algebra of the kernel of a homomorphism 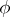 of analytic groups is the kernel of the homomorphism
of analytic groups is the kernel of the homomorphism 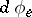 of their Lie algebras. The Lie algebra of the quotient group
of their Lie algebras. The Lie algebra of the quotient group 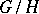 , where
, where  is an analytic normal subgroup of
is an analytic normal subgroup of 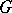 , is the quotient algebra of the Lie algebra of
, is the quotient algebra of the Lie algebra of 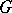 with respect to the ideal corresponding to
with respect to the ideal corresponding to 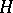 . If
. If  is the Lie algebra of an analytic group
is the Lie algebra of an analytic group 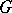 and
and  is a subalgebra of
is a subalgebra of  , then there is a unique connected Lie subgroup
, then there is a unique connected Lie subgroup  with Lie algebra
with Lie algebra  ;
; 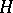 need not be closed in
need not be closed in 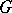 . The Lie algebra of an analytic group is solvable (nilpotent, semi-simple) if and only if the group itself is solvable (nilpotent, semi-simple).
. The Lie algebra of an analytic group is solvable (nilpotent, semi-simple) if and only if the group itself is solvable (nilpotent, semi-simple).
This connection between the categories of analytic groups and Lie algebras is not, however, an equivalence of these categories, in contrast to the case of local Lie groups. Namely, non-isomorphic analytic groups can have isomorphic Lie algebras. Analytic groups with isomorphic Lie algebras are said to be locally isomorphic. In the case of a field 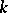 of characteristic zero, to each finite-dimensional Lie algebra over
of characteristic zero, to each finite-dimensional Lie algebra over 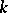 corresponds a class of locally isomorphic analytic groups. Suppose that
corresponds a class of locally isomorphic analytic groups. Suppose that 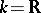 or
or 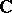 . Among all locally isomorphic analytic groups there is a connected simply-connected group, which is unique up to isomorphism; the category of analytic groups of this type is equivalent to the category of finite-dimensional Lie algebras over
. Among all locally isomorphic analytic groups there is a connected simply-connected group, which is unique up to isomorphism; the category of analytic groups of this type is equivalent to the category of finite-dimensional Lie algebras over 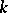 . In particular, every homomorphism of Lie algebras is induced by an analytic homomorphism of the corresponding connected simply-connected analytic groups. Any connected Lie group that is locally isomorphic to a given connected simply-connected Lie group
. In particular, every homomorphism of Lie algebras is induced by an analytic homomorphism of the corresponding connected simply-connected analytic groups. Any connected Lie group that is locally isomorphic to a given connected simply-connected Lie group  has the form
has the form 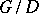 , where
, where 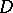 is a discrete normal subgroup lying in the centre of
is a discrete normal subgroup lying in the centre of 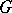 .
.
References
| [1] | N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French) |
| [2] | L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1958) (Translated from Russian) |
| [3] | J.-P. Serre, "Lie algebras and Lie groups" , Benjamin (1965) (Translated from French) |
| [4] | A.A. Kirillov, "Elements of the theory of representations" , Springer (1976) (Translated from Russian) |
| [5] | C. Chevalley, "Theory of Lie groups" , 1 , Princeton Univ. Press (1946) |
Comments
The unique connected simply-connected Lie group over 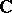 or
or 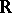 that is locally isomorphic to a connected Lie group
that is locally isomorphic to a connected Lie group 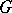 is called the covering group of
is called the covering group of 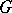 . Existence and uniqueness are due to L.S. Pontryagin (1966).
. Existence and uniqueness are due to L.S. Pontryagin (1966).
So, the global structure of a Lie group 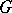 is as follows.
is as follows. 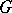 consists of a discrete number of connected components. The component
consists of a discrete number of connected components. The component 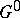 containing the identity is normal in
containing the identity is normal in 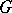 (and both open and closed). Thus
(and both open and closed). Thus 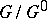 is a discrete group. Often, particularly when
is a discrete group. Often, particularly when 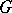 is compact,
is compact, 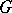 is a semi-direct product:
is a semi-direct product: 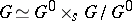 . Finally, there exists a simply-connected connected covering group
. Finally, there exists a simply-connected connected covering group  of
of 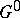 with a projection
with a projection 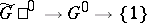 of which the kernel is discrete and contained in the centre of
of which the kernel is discrete and contained in the centre of  .
.
References
| [a1] | V.S. Varadarajan, "Lie groups, Lie algebras and their representations" , Prentice-Hall (1974) |
Lie algebra of an analytic group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lie_algebra_of_an_analytic_group&oldid=13500