Scalar curvature
From Encyclopedia of Mathematics
of a Riemannian manifold at a point 
The trace of the Ricci tensor with respect to the metric tensor 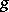 . The scalar curvature
. The scalar curvature  is connected with the Ricci curvature
is connected with the Ricci curvature  and the sectional curvature
and the sectional curvature  by the formulas
by the formulas
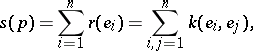 |
where  is an orthonormal basis of the tangent space. In the equivalent Einstein notation, these equations have the form
is an orthonormal basis of the tangent space. In the equivalent Einstein notation, these equations have the form
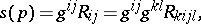 |
where 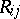 and
and  are the components of the Ricci tensor and the curvature tensor, respectively, and the
are the components of the Ricci tensor and the curvature tensor, respectively, and the 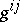 are the contravariant components of the metric tensor.
are the contravariant components of the metric tensor.
References
| [1] | D. Gromoll, W. Klingenberg, W. Meyer, "Riemannsche Geometrie im Grossen" , Springer (1968) |
| [2] | P.K. [P.K. Rashevskii] Rashewski, "Riemannsche Geometrie und Tensoranalyse" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian) |
Comments
References
| [a1] | S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 1–2 , Interscience (1963–1969) |
How to Cite This Entry:
Scalar curvature. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Scalar_curvature&oldid=12835
Scalar curvature. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Scalar_curvature&oldid=12835
This article was adapted from an original article by L.A. Sidorov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article