Continuous series of representations

principal series of representations

The family of irreducible unitary representations of a locally compact group 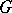that occur in the decomposition of the regular representation of , but do not belong to the discrete series (of representations) of this group. If is a real semi-simple Lie group, 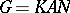is its Iwasawa decomposition and 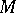is the centralizer of 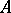in 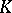, then the non-degenerate continuous principal series of representations of 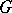is the family of irreducible unitary representations of induced by the finite-dimensional irreducible unitary representations of the group that are trivial on .

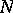The complementary (or degenerate) continuous series of representations of such a group 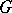is the family of irreducible unitary representations of 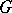that occur in the complementary series (of representations) (respectively, the degenerate series of representations) of 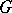and are not isolated points of it (as subsets of the dual space of 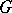). The analytic continuation of the non-degenerate continuous principal series of representations is the family of (generally speaking, non-unitary) representations of induced by all possible finite-dimensional irreducible representations of 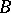that are trivial on 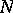. This family plays a decisive role in the representation theory of real semi-simple Lie algebras and harmonic analysis on these groups; in particular, any completely irreducible representation of in a Hilbert space is infinitesimally equivalent to a subrepresentation of some quotient representation of one of the representations of the analytic continuation of the non-degenerate continuous principal series of representations. See also Infinite-dimensional representation of a Lie group.

References

[1]	I.M. Gel'fand, M.A. Naimark, "Unitäre Darstellungen der klassischen Gruppen" , Akademie Verlag (1957) (Translated from Russian)
[2]	A.A. Kirillov, "Elements of the theory of representations" , Springer (1976) (Translated from Russian)
[3]	G. Warner, "Harmonic analysis on semi-simple Lie groups" , 1–2 , Springer (1972)

Comments

In the Western literature one customarily uses the terminology principal series instead of non-degenerate continuous principal series. Note that 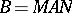as above is a minimal parabolic subgroup. One even speaks of a (generalized) principal series representation in the following situation. Let be a connected semi-simple matrix group and let be a cuspidal (not necessarily minimal) parabolic subgroup. Fix a Langlands decomposition 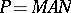with 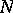the unipotent radical and 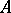a vector group. Let 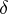be a discrete series representation of 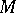and a (non-unitary) character of 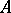. The induced representation

is called a generalized principal series representation. When is unitary, these are the representations occurring in Harish-Chandra's Plancherel formula for . The result mentioned at the end of the entry above usually is called the subquotient theorem: Any irreducible admissible representation of can be realized canonically as a subquotient of a generalized principal series representation.

References

[a1]	A.W. Knopp, "Representation of semisimple groups" , Princeton Univ. Press (1986)
[a2]	D.A. Vogan jr., "Representations of real reductive Lie groups" , Birkhäuser (1981)