Chebyshev approximation

uniform approximation

Approximation of a continuous function 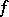defined on a set by functions from a given class of functions, where the measure of approximation is the deviation in the uniform metric

P.L. Chebyshev in 1853 [1] raised and studied the problem of best uniform approximation of a continuous function by algebraic polynomials of degree not exceeding . For this problem, and also for the more general problem concerning best uniform approximation of a function by rational functions, he obtained fundamental results, and at the same time laid the foundations for the theory of best approximation.

References

[1]	P.L. Chebyshev, , Collected works , 2 , Moscow-Leningrad (1947) pp. 23–51 (In Russian)
[2]	R.S. Guter, L.D. Kudryavtsev, B.M. Levitan, "Elements of the theory of functions" , Moscow (1963) (In Russian)

Comments

See also [a1], especially Chapt. 3, and [a2], Section 7.6. For an obvious reason, Chebyshev approximation is also called best uniform approximation.

References

[a1]	E.W. Cheney, "Introduction to approximation theory" , McGraw-Hill (1966)
[a2]	P.J. Davis, "Interpolation and approximation" , Dover, reprint (1975) pp. 108–126