Luzin-Privalov theorems
in the theory of functions of a complex variable
Classical results of N.N. Luzin and I.I. Privalov that clarify the character of a boundary uniqueness property of analytic functions (cf. Uniqueness properties of analytic functions) (see [1]).
1) Let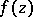 
be a meromorphic function of the complex variable 
in a simply-connected domain 
with rectifiable boundary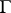 
. If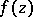 
takes angular boundary values zero on a set 
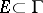of positive Lebesgue measure on 
, then 
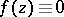in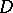 
. There is no function meromorphic in 
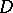that has infinite angular boundary values on a set 
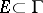of positive measure.
2) Let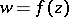 
be a meromorphic function in the unit disc 
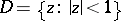other than a constant and having radial boundary values (finite or infinite) on a set 
situated on an arc 
of the unit circle 
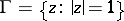that is metrically dense and of the second Baire category (cf. Baire classes) on 
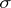. Then the set 
of its radial boundary values on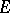 
contains at least two distinct points. Metric density of 
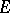on 
means that every portion of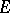 
on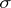 
has positive measure. This implies that if the radial boundary values of 
on a set 
of the given type are equal to zero, then 
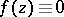in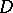 
. Moreover, there is no meromorphic function in the unit disc that takes infinite radial boundary values on a set 
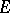of the given type.
Luzin and Privalov (see [1], [2]) constructed examples to show that neither metric density nor second Baire category are by themselves sufficient for the assertion in 2 to hold.
See also Boundary properties of analytic functions; Luzin examples; Cluster set; Privalov theorem; Riesz theorem.
References
| [1] | N.N. [N.N. Luzin] Lusin, I.I. [I.I. Privalov] Priwaloff, "Sur l'unicité et la multiplicité des fonctions analytiques" Ann. Sci. Ecole Norm. Sup. (3) , 42 (1925) pp. 143–191 |
| [2] | I.I. [I.I. Privalov] Priwalow, "Randeigenschaften analytischer Funktionen" , Deutsch. Verlag Wissenschaft. (1956) (Translated from Russian) |
| [3] | A. Lohwater, "The boundary behaviour of analytic functions" Itogi Nauk. i Tekhn. Mat. Anal. , 10 (1973) pp. 99–259 (In Russian) |
Comments
References
| [a1] | E.F. Collingwood, A.J. Lohwater, "The theory of cluster sets" , Cambridge Univ. Press (1966) pp. Chapt. 9 |
Luzin-Privalov theorems. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Luzin-Privalov_theorems&oldid=12447