Characteristic exponent

The same as a Lyapunov characteristic exponent.

The characteristic exponents of a linear system of ordinary differential equations with periodic coefficients are the quotients on division of the natural logarithms of the multipliers of the system by the period of the coefficients of the system. In this case the Lyapunov characteristic exponents of the system are equal to the real parts of the characteristic exponents of this system. An equivalent definition is: A number 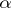is called a characteristic exponent of a linear system of ordinary differential equations with periodic coefficients if this system has a complex solution of the form 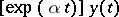, where the vector function , , 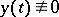is periodic in 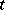with the same period, 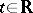, and .

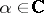The expression "characteristic exponent of a solution of a system of ordinary differential equationscharacteristic exponent of a solution of a system of ordinary differential equations" also occurs when the system in question is non-linear. By this expression one means a characteristic exponent of the system of equations in variations of the given system along a given solution, where in turn the term "characteristic exponent" can be understood in the sense of 1) or 2).

References

[a1]	V.V. Nemytskii, V.V. Stepanov, "Qualitative theory of differential equations" , Princeton Univ. Press (1960) (Translated from Russian)