Birkhoff stratification

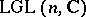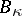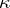As an immediate consequence of Birkhoff factorization, [a1], the group of differentiable invertible matrix loops may be decomposed in a union of subsets , labelled by unordered -tuples of integers . Each of these consists of all loops with 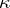as the set of partial indices. This decomposition is called a Birkhoff stratification. It reflects important properties of holomorphic vector bundles over the Riemann sphere [a2], singular integral equations [a3], and Riemann–Hilbert problems [a4]. The structure of a Birkhoff stratification resembles those of Schubert decompositions of Grassmannians and Bruhat decompositions of complex Lie groups (cf. also Bruhat decomposition). The Birkhoff strata are complex submanifolds of finite codimension in 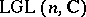. Codimension, homotopy type and cohomological fundamental class of 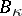are expressible in terms of the label 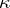[a5]. The adjacencies among the Birkhoff strata describe deformations of holomorphic vector bundles [a5]. Birkhoff stratifications also exist for loop groups of compact Lie groups [a6]. For the group of based loops 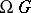on a compact Lie group 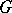, the Birkhoff strata are contractible complex submanifolds labelled by the conjugacy classes of homomorphisms 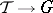[a6]. Birkhoff stratification has a visual interpretation in the framework of Morse theory of the energy function on 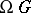[a6]. Certain geometric aspects of Birkhoff stratification may be described in terms of non-commutative differential geometry and Fredholm structures [a7], [a8]. In particular, the Birkhoff strata become Fredholm submanifolds of 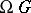endowed with various Fredholm structures. Fredholm structures on arise from the natural Kähler structure on [a7] and in the context of generalized Riemann–Hilbert problems with coefficients in 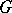[a8]. Curvatures and characteristic classes of Birkhoff strata may be computed in the spirit of non-commutative differential geometry, in terms of regularized traces of appropriate Toeplitz operators [a7].

References

[a1]	G.D. Birkhoff, "Singular points of ordinary linear differential equations" Trans. Amer. Math. Soc. , 10 (1909) pp. 436–470
[a2]	A. Grothendieck, "Sur la classification des fibrés holomorphes sur la sphère de Riemann" Amer. J. Math. , 79 (1957) pp. 121–138
[a3]	I.Z. Gohberg, M.G. Krein, "Systems of integral equations on a half-line with kernels depending on the difference of the arguments" Transl. Amer. Math. Soc. , 14 (1960) pp. 217–284
[a4]	B. Bojarski, "On the stability of Hilbert problem for holomorphic vector" Bull. Acad. Sci. Georgian SSR , 21 (1958) pp. 391–398
[a5]	S. Disney, "The exponents of loops on the complex general linear group" Topology , 12 (1973) pp. 297–315
[a6]	A. Pressley, G. Segal, "Loop groups" , Clarendon Press (1986)
[a7]	D. Freed, "The geometry of loop groups" J. Diff. Geom. , 28 (1988) pp. 223–276
[a8]	G. Khimshiashvili, "Lie groups and transmission problems on Riemann surfaces" Contemp. Math. , 131 (1992) pp. 164–178