Contracting-mapping principle

contractive-mapping principle, contraction-mapping principle

A theorem asserting the existence and uniqueness of a fixed point of a mapping 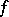of a complete metric space (or a closed subset of such a space) into itself, if for any the inequality

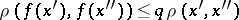(1)

holds, for some fixed , 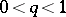. This principle is widely used in the proof of the existence and uniqueness of solutions not only of equations of the form 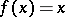, but also of equations 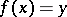, by changing the equation to the equivalent: , where .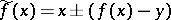

The scheme of application of the principle is usually as follows: Starting from the properties of 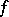first find a closed set 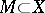, usually a closed ball, such that 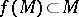, and then prove that on this set is a contractive mapping. After this, starting from an arbitrary element 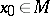, construct the sequence , , 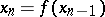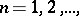belonging to 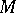, which converges to some element . This will be the unique solution of the equation , and will be a sequence of approximate solutions.

In general, condition (1) cannot be changed to

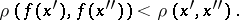(2)

However, if this condition is satisfied on a compact set 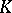that is mapped into itself by , then it guarantees the existence of a unique fixed point 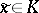for .

The following generalization of the contractive-mapping principle holds. Again, let map a complete metric space 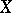into itself and let

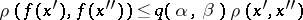for 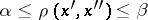, where 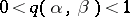for . Then 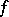has a unique fixed point in .

References

[1]	A.N. Kolmogorov, S.V. Fomin, "Elements of the theory of functions and functional analysis" , 1–2 , Graylock (1957–1961) (Translated from Russian)
[2]	M.A. Krasnosel'skii, G.M. Vainikko, P.P. Zabreiko, et al., "Approximate solution of operator equations" , Wolters-Noordhoff (1972) (Translated from Russian)
[3]	L.A. Lyusternik, V.I. Sobolev, "Elements of functional analysis" , Hindushtan Publ. Comp. (1974) (Translated from Russian)
[4]	V. Trenogin, "Functional analysis" , Moscow (1980) (In Russian)

Comments

This principle is also known as the contraction principle or Banach's fixed-point theorem. It was proved by S. Banach in [a1]. The generalization discussed at the end of the article above goes by the name generalized contraction mapping in the sense of Krasnosel'skii [a5], [a6]. For this and other generalizations of the idea of a contractive mapping, cf. [a4], Chapt. 3.

References

[a1]	S. Banach, "Sur les opérations dans les ensembles abstraits et leurs application aux équations intégrales" Fund. Math. , 3 (1922) pp. 7–33
[a2]	W. Rudin, "Principles of mathematical analysis" , McGraw-Hill (1953)
[a3]	S. Willard, "General topology" , Addison-Wesley (1970)
[a4]	V.I. Istrăţescu, "Fixed point theory" , Reidel (1981)
[a5]	M.A. Krasnosel'skii, "Topological methods in the theory of nonlinear integral equations" , Macmillan (1964) (Translated from Russian)
[a6]	M.A. Krasnosel'skii, "Positive solutions of operator equations" , Noordhoff (1964) (Translated from Russian)