Schur index
The Schur index of a central simple algebra  over a field
over a field  (cf. Central simple algebra) is the degree of the division algebra
(cf. Central simple algebra) is the degree of the division algebra  such that
such that 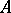 is a full matrix algebra
is a full matrix algebra 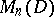 over
over  .
.
Let 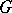 be a finite group,
be a finite group, 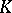 a field and
a field and  the algebraic closure of
the algebraic closure of 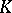 . Let
. Let 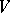 be an irreducible
be an irreducible 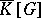 -module with character
-module with character 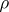 (cf. Irreducible module). Let
(cf. Irreducible module). Let  be obtained from
be obtained from 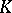 by adjoining the values
by adjoining the values 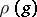 ,
, 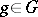 . The Schur index of the module
. The Schur index of the module 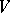 ,
, 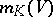 , (or the Schur index of the character
, (or the Schur index of the character 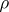 ) is the minimal degree of a field
) is the minimal degree of a field 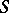 extending
extending 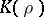 such that
such that 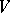 descends to
descends to 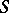 , i.e. such that there is an
, i.e. such that there is an 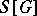 -module
-module  for which
for which  .
.
For a finite field 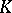 the Schur index is always
the Schur index is always 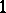 , [a1].
, [a1].
A basic result on the Schur index is that for each 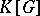 -module
-module 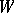 the multiplicity of
the multiplicity of  in
in  is a multiple of
is a multiple of 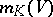 .
.
A field 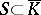 is a splitting field for a finite group
is a splitting field for a finite group 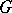 if each irreducible
if each irreducible 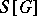 -module is absolutely irreducible, i.e. if
-module is absolutely irreducible, i.e. if 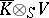 is irreducible. The basic result on the Schur index quoted above readily leads to a proof of R. Brauer's result [a1] that if
is irreducible. The basic result on the Schur index quoted above readily leads to a proof of R. Brauer's result [a1] that if 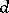 is the exponent of a finite group
is the exponent of a finite group 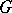 (i.e.
(i.e. 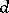 is the smallest integer such that
is the smallest integer such that 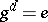 for all
for all 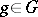 ), then
), then 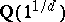 is a splitting field for
is a splitting field for 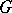 .
.
The set 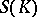 of classes of central simple algebras over
of classes of central simple algebras over 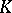 which occur as components of a group algebra
which occur as components of a group algebra 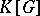 for some finite group
for some finite group 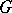 is a subgroup of the Brauer group
is a subgroup of the Brauer group 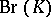 of
of  , and is known as the Schur subgroup of
, and is known as the Schur subgroup of 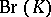 . Cf. [a4] for results on the structure of
. Cf. [a4] for results on the structure of 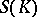 .
.
References
| [a1] | R. Brauer, "On the representation of a group of order  in the field of in the field of 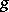 -th roots of unity" Amer. J. Math. , 67 (1945) pp. 461–471 -th roots of unity" Amer. J. Math. , 67 (1945) pp. 461–471 |
| [a2] | C.W. Curtis, I. Reiner, "Representation theory of finite groups and associative algebras" , Interscience (1962) pp. §90, §41 |
| [a3] | B. Huppert, "Finite groups" , 2 , Springer (1982) pp. §1 |
| [a4] | T. Yamada, "The Schur subgroup of the Brauer group" , Springer (1974) |
Schur index. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Schur_index&oldid=11986