Riesz decomposition property
Let  be a partially ordered vector space, [a5], i.e.
be a partially ordered vector space, [a5], i.e.  is a real vector space with a convex cone
is a real vector space with a convex cone  defining the partial order by
defining the partial order by  if and only if
if and only if  . For
. For  , the corresponding interval is
, the corresponding interval is  .
.
The (partially) ordered vector space  has the Riesz decomposition property if
has the Riesz decomposition property if  for all
for all  , or, equivalently, if
, or, equivalently, if  for all
for all  ,
, 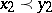 .
.
A Riesz space (or vector lattice) automatically has the Riesz decomposition property.
Terminology on this concept varies a bit: in [a2] the property is referred to as the dominated decomposition property, while in [a3] it is called the decomposition property of F. Riesz.
The Riesz decomposition property and the Riesz decomposition theorem are quite different (although there is a link) and, in fact, the property does turn up as an axiom used in axiomatic potential theory (see also Potential theory, abstract), see [a1], where it is called the axiom of natural decomposition.
There is a natural non-commutative generalization to the setting of  -algebras, as follows, [a4]. Let
-algebras, as follows, [a4]. Let  ,
, 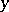 ,
,  be elements of a
be elements of a  -algebra
-algebra  . If
. If  , then there are
, then there are  such that
such that 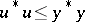 ,
, 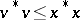 and
and  .
.
References
| [a1] | C. Constantinescu, A. Cornea, "Potential theory on harmonic spaces" , Springer (1972) pp. 104 |
| [a2] | W.A.J. Luxemburg, A.C. Zaanen, "Riesz spaces" , I , North-Holland (1971) pp. 73 |
| [a3] | P. Meyer-Nieberg, "Banach lattices" , Springer (1971) pp. 3, Thm. 1.1.1 |
| [a4] | G.K. Pedersen, "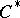 -algebras and their automorphism groups" , Acad. Press (1979) pp. 14 -algebras and their automorphism groups" , Acad. Press (1979) pp. 14 |
| [a5] | Y.-Ch. Wong, K.-F. Ng, "Partially ordered topological vector spaces" , Oxford Univ. Press (1973) pp. 9 |
Riesz decomposition property. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Riesz_decomposition_property&oldid=50384