Stieltjes polynomials
A system of polynomials  which satisfy the orthogonality condition
which satisfy the orthogonality condition
 |
where the weight function  satisfies
satisfies  ,
,  , with finite moments
, with finite moments  .
.  is the system of orthogonal polynomials associated with
is the system of orthogonal polynomials associated with  . The degree of
. The degree of  is equal to its index
is equal to its index 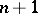 . The orthogonality conditions define
. The orthogonality conditions define  up to a multiplicative constant, but the conditions for
up to a multiplicative constant, but the conditions for 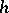 given above are not sufficient for
given above are not sufficient for 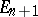 to have real zeros in
to have real zeros in 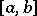 . However, several special cases and classes of weight functions
. However, several special cases and classes of weight functions  are known for which the zeros of the corresponding Stieltjes polynomials do not only have this property, but also interlace with the zeros of
are known for which the zeros of the corresponding Stieltjes polynomials do not only have this property, but also interlace with the zeros of 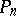 . A simple example is
. A simple example is  ,
, 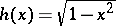 ,
, 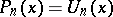 , the Chebyshev polynomial of the second kind (cf. Chebyshev polynomials), where
, the Chebyshev polynomial of the second kind (cf. Chebyshev polynomials), where  , the Chebyshev polynomial of the first kind. For
, the Chebyshev polynomial of the first kind. For 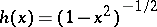 ,
,  and
and  . A generalization are the Bernstein–Szegö weight functions
. A generalization are the Bernstein–Szegö weight functions
 |
where 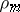 is a polynomial of degree
is a polynomial of degree  that is positive in
that is positive in  [a6], [a7]. Weight functions for which
[a6], [a7]. Weight functions for which 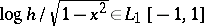 ,
, 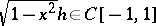 and
and 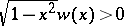 for
for  are another class for which the above properties are known to hold asymptotically under certain additional conditions on
are another class for which the above properties are known to hold asymptotically under certain additional conditions on 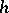 [a8].
[a8].
The classical case originally considered by Th.J. Stieltjes is the Legendre weight function  ,
, 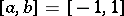 . For this case G. Szegö [a9] proved that all zeros are real, belong to the open interval
. For this case G. Szegö [a9] proved that all zeros are real, belong to the open interval 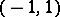 and interlace with the zeros of the Legendre polynomials
and interlace with the zeros of the Legendre polynomials  . Szegö extended his proof to the ultraspherical, or Gegenbauer, weight function,
. Szegö extended his proof to the ultraspherical, or Gegenbauer, weight function, 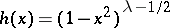 ,
, 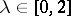 ,
,  , cf. also Gegenbauer polynomials; Ultraspherical polynomials. For the more general Jacobi weight
, cf. also Gegenbauer polynomials; Ultraspherical polynomials. For the more general Jacobi weight 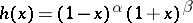 , results of existence and non-existence can be found in [a4]. Comparatively little is known for unbounded intervals. Numerical results reported in [a5] show that complex zeros arise for the Laguerre weight
, results of existence and non-existence can be found in [a4]. Comparatively little is known for unbounded intervals. Numerical results reported in [a5] show that complex zeros arise for the Laguerre weight  ,
,  ,
,  and the Hermite weight
and the Hermite weight 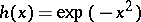 ,
, 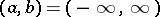 .
.
An important fact for the analysis of the Stieltjes polynomials  is their close connection with the functions of the second kind
is their close connection with the functions of the second kind 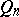 associated with
associated with 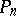 and
and 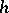 . Stieltjes [a1] proved that
. Stieltjes [a1] proved that 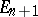 is precisely the polynomial part of the Laurent expansion of
is precisely the polynomial part of the Laurent expansion of  . Szegö's work in [a9] and subsequent investigations are based on this connection.
. Szegö's work in [a9] and subsequent investigations are based on this connection.
Several asymptotic representations are available. A simple formula for the Legendre weight function is
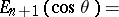 |
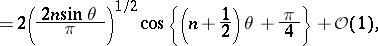 |
uniformly for  , see [a2]. Inequalities for Stieltjes polynomials in the case
, see [a2]. Inequalities for Stieltjes polynomials in the case  can be found in [a3].
can be found in [a3].
The zeros of Stieltjes polynomials are used for quadrature and for interpolation. In particular, the often-used Gauss–Kronrod quadrature formulas (cf. Gauss–Kronrod quadrature formula) are based on the union of the zeros of 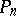 and
and 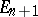 and enable an efficient estimation for the Gauss quadrature formula based on the zeros of
and enable an efficient estimation for the Gauss quadrature formula based on the zeros of 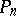 . This idea has been carried over to extended interpolation processes (cf. Extended interpolation process). For
. This idea has been carried over to extended interpolation processes (cf. Extended interpolation process). For  , adding the zeros of
, adding the zeros of  improves the interpolation process based on the zeros of
improves the interpolation process based on the zeros of  to an optimal-order interpolation process [a3] (see also Extended interpolation process).
to an optimal-order interpolation process [a3] (see also Extended interpolation process).
References
| [a1] | B. Baillaud, H. Bourget, "Correspondance d'Hermite et de Stieltjes" , I,II , Gauthier-Villars (1905) |
| [a2] | S. Ehrich, "Asymptotic properties of Stieltjes polynomials and Gauss–Kronrod quadrature formulae" J. Approx. Th. , 82 (1995) pp. 287–303 |
| [a3] | S. Ehrich, G. Mastroianni, "Stieltjes polynomials and Lagrange interpolation" Math. Comput. , 66 (1997) pp. 311–331 |
| [a4] | W. Gautschi, S.E. Notaris, "An algebraic study of Gauss–Kronrod quadrature formulae for Jacobi weight functions" Math. Comput. , 51 (1988) pp. 231–248 |
| [a5] | G. Monegato, "Stieltjes polynomials and related quadrature rules" SIAM Review , 24 (1982) pp. 137–158 |
| [a6] | S.E. Notaris, "Gauss–Kronrod quadrature for weight functions of Bernstein–Szegö type" J. Comput. Appl. Math. , 29 (1990) pp. 161–169 |
| [a7] | F. Peherstorfer, "Weight functions admitting repeated positive Kronrod quadrature" BIT , 30 (1990) pp. 241–251 |
| [a8] | F. Peherstorfer, "Stieltjes polynomials and functions of the second kind" J. Comput. Appl. Math. , 65 (1995) pp. 319–338 |
| [a9] | G. Szegö, "Über gewisse orthogonale Polynome, die zu einer oszillierenden Belegungsfunktion gehören" Math. Ann. , 110 (1934) pp. 501–513 (Collected papers, Vol.2, R. Askey (Ed.), Birkhäuser, 1982, 545-557) |
Stieltjes polynomials. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Stieltjes_polynomials&oldid=50350