Fredholm spectrum

A bounded operator on a complex Hilbert space is said to be essentially left invertible (respectively, essentially right invertible) if there exists a bounded operator such that (respectively, ) has finite rank. An operator that is essentially left or right invertible is also called a semi-Fredholm operator, and is a Fredholm operator if it is both left and right essentially invertible. F.V. Atkinson proved that an operator is Fredholm if and only if it has closed range and the spaces and have finite dimension. For a semi-Fredholm operator one defines the index

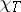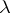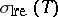this is an integer or . The set of semi-Fredholm operators is open, and the function is continuous (thus locally constant). Moreover, if is a compact operator; is finite if is Fredholm. Let be the set of complex scalars such that is not semi-Fredholm; is a closed subset of 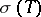(cf. Spectrum of an operator). The difference 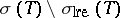is called the semi-Fredholm spectrum of , and a value in this set belongs to the Fredholm spectrum of if 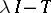is Fredholm. If 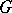is one of the connected components of 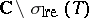, then the number 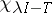is constant for ; call it 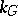. If 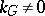, then is entirely contained in the semi-Fredholm spectrum of 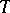(in the Fredholm spectrum i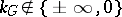f ). If , then either 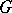is contained in the Fredholm spectrum of 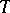, or 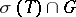has no accumulation points in 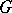. More generally, I.C. Gohberg showed that 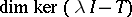is constant for 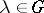, with the possible exception of a countable set with no accumulation points in ; the dimension is greater if is one of the exceptional points.

An interesting calculation of the Fredholm spectrum was done by Gohberg when is a Toeplitz operator (cf. also Toeplitz matrix; Calderón–Toeplitz operator) whose symbol is a continuous function on the unit circle. In this case 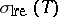is the range of 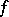, and equals minus the winding number of about if .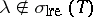

The presence of a semi-Fredholm spectrum is related with the notion of quasi-triangularity introduced by P.R. Halmos. An operator 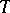is quasi-triangular if it can be written as where 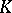is compact and is triangular in some basis. R.G. Douglas and C.M. Pearcy showed that cannot be quasi-triangular if 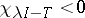for some in the semi-Fredholm spectrum of . Quite surprisingly, the converse of this statement is also true, as shown by C. Apostol, C. Foiaş and D. Voiculescu. Subsequent developments involving the Fredholm spectrum include the approximation theory of Hilbert-space operators developed by Apostol, D. Herrero, and Voiculescu.

The notion of Fredholm spectrum can be extended to operators on other topological vector spaces, to unbounded operators, and to -tuples of commuting operators. A different generalization is to define the Fredholm spectrum for elements in von Neumann algebras. The algebra of bounded operators on a Hilbert space is a factor of type 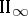. An appropriate notion of finite-rank element exists in any factor of type 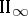, and this leads to a corresponding notion of Fredholm operator and Fredholm spectrum. Analogues have been found in other Banach algebras as well.

The Fredholm property was also defined in a non-linear context by S. Smale. A differentiable mapping (cf. also Differentiation of a mapping) between two open sets in a Banach space is Fredholm if its derivative at every point is a linear Fredholm operator. This leads to the notion of a Fredholm mapping on an infinite-dimensional manifold. Smale used this notion to extend to infinite dimensions certain results of A. Sard and R. Thom.