Tilting functor

When studying an algebra , it is sometimes convenient to consider another algebra, given for instance by the endomorphism of an appropriate -module, and functors between the two module categories. For instance, this is the basis of the Morita equivalence or the construction of the so-called Auslander algebras. An important example of this strategy is given by the tilting theory and the tilting functors, as now described.

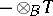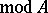Let be a finite-dimensional -algebra, where is a field, a tilting (finitely-generated) -module (cf. Tilting module) and . One can then assign to the functors , , , and , which are called tilting functors. The importance of considering such functors is that they give equivalences between subcategories of the module categories and 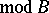, results first established by S. Brenner and M.C.R. Butler. Namely, and its adjoint give an equivalence between the subcategories

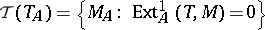and

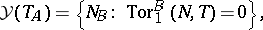while and give an equivalence between the subcategories

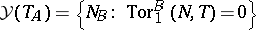and

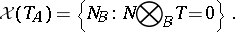It is not difficult to see that and 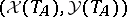are torsion pairs in and , respectively. Clearly, one can now transfer information from to . One of the most interesting cases occurs when is a hereditary algebra and so the torsion pair splits, giving in particular that each indecomposable -module is the image of an indecomposable -module either by or by 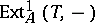(in this case, the algebra is called tilted, cf. also Tilted algebra).

This procedure has been generalized in several ways and it is worthwhile mentioning, for instance, its connection with derived categories (cf. also Derived category), or the notion of quasi-tilted algebras. It has also been considered for infinitely-generated modules over arbitrary rings.

For referenes, see also Tilting theory; Tilted algebra.