Variational problem
A variational problem with fixed ends is a problem in variational calculus in which the end points of the curve which gives the extremum are fixed. Thus, in the simplest problem in variational calculus, 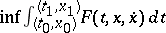 with fixed ends, the initial and final points
with fixed ends, the initial and final points 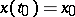 ,
, 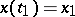 through which the sought curve
through which the sought curve 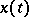 should pass are given. Since the general solution of the Euler equation of the simplest problem depends on two arbitrary constants,
should pass are given. Since the general solution of the Euler equation of the simplest problem depends on two arbitrary constants, 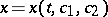 , the curve giving the extremum will be found among the solutions of the corresponding boundary value problem. It may turn out that the boundary value problem has only one solution, more than one solution or no solution at all.
, the curve giving the extremum will be found among the solutions of the corresponding boundary value problem. It may turn out that the boundary value problem has only one solution, more than one solution or no solution at all.
A variational problem with free (mobile) ends is a problem in variational calculus in which the end points of the curve which gives the extremum may move along given manifolds. For instance, if in the Bolza problem the number of boundary conditions to be satisfied by the sought curve 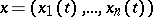 is strictly less than
is strictly less than 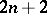 :
:
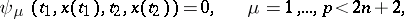 | (*) |
the end points of the curve may move along the 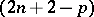 -dimensional manifold (*). If the boundary conditions (*) are given in the form
-dimensional manifold (*). If the boundary conditions (*) are given in the form
 |
 |
and 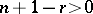 or
or 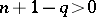 , the end points of the curve
, the end points of the curve 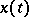 may move along the respective manifolds of dimensions
may move along the respective manifolds of dimensions 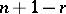 or
or 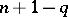 . At the end points of the extremal curve the transversality condition must be met; this, together with the conditions (*), makes it possible to obtain a closed system of relations leading to some boundary value problem. The solution of this boundary value problem yields arbitrary constants, which appear in the general integral of the Euler equation.
. At the end points of the extremal curve the transversality condition must be met; this, together with the conditions (*), makes it possible to obtain a closed system of relations leading to some boundary value problem. The solution of this boundary value problem yields arbitrary constants, which appear in the general integral of the Euler equation.
The qualitative difference between variational problems and the problem of finding extrema of a function of several variables consists in the fact that in the former case one is looking not for a point in a finite-dimensional space, but for a function (or a point in an infinite-dimensional space).
Comments
References
| [a1] | A.R.M. Noton, "Introduction to variational methods in control engineering" , Pergamon (1965) Zbl 0145.34101 |
| [a2] | W.H. Fleming, R.W. Rishel, "Deterministic and stochastic optimal control" , Springer (1975) MR0454768 Zbl 0323.49001 |
| [a3] | L. Cesari, "Optimization - Theory and applications" , Springer (1983) MR0688142 Zbl 0506.49001 |
| [a4] | N.I. Akhiezer, "The calculus of variations" , Blaisdell (1962) (Translated from Russian) MR0142019 Zbl 0718.49001 |
Variational problem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Variational_problem&oldid=49126