Two-dimensional knot
A class of isotopic imbeddings of the two-dimensional sphere 
in the four-dimensional one 
. The condition of local planarity is usually imposed. The method of study consists in considering sections of 
by a bundle of three-dimensional parallel planes. The fundamental problem is whether or not the knot will be trivial if its group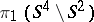 
is isomorphic to 
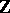. It is known that in such a case the complement 
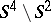has the homotopy type of 
.
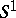A 
-ribbon in 
is the image 
of an immersion 
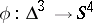, where 
is the three-dimensional disc, such that: 1)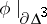 
is an imbedding; 2) the self-intersection of 
consists of a finite number of pairwise non-intersecting two-dimensional discs 
; and 3) the pre-image 
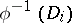of each disc 
is a union of two discs 
and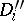 
such that
 |
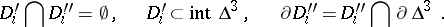The image of the boundary 
is a two-dimensional knot in 
. The knots thus obtained are said to be ribbon knots. This is one of the most thoroughly studied class of two-dimensional knots. Any two-dimensional ribbon knot is the boundary of some three-dimensional submanifold of the sphere 
which is homeomorphic either to the disc 
or to the connected sum of some number of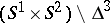 
. A two-dimensional ribbon knot is trivial if and only if the fundamental group of its complement is isomorphic to 
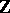. A group 
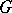is the group of some two-dimensional ribbon knot in 
if and only if it has a Wirtinger presentation (i.e. a presentation 
, where each relation has the form 
) in which the number of relations is one smaller than the number of generators and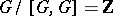 
.
The class of groups of all two-dimensional knots has not yet been fully described. It is known that this class is wider than that of the one-dimensional knots in 
but smaller than the class of groups of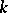 
-dimensional knots in 
, 
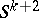. The latter class has been fully characterized (cf. Multi-dimensional knot). The following properties are displayed by two-dimensional knot groups (but not, in general, by the groups of three-dimensional knots in 
):
 |
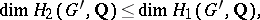where 
is the commutator subgroup; on the finite group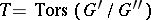 
there exists a non-degenerate symmetric form 
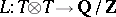such that for any 
, 
one has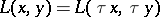 
, where 
is the automorphism induced in 
by conjugation with the element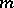 
.
The calculation of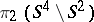 
has been done only for special types of two-dimensional knots, e.g. those obtained by Artin's construction, ribbon knots and fibred knots.
Two-dimensional knot. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Two-dimensional_knot&oldid=49051