Strong topology
A dual pair of vector spaces 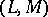 over a field
over a field 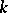 is a pair of vector spaces
is a pair of vector spaces 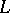 ,
, 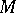 together with a non-degenerate bilinear form over
together with a non-degenerate bilinear form over  ,
,
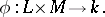 |
I.e. 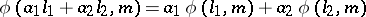 ,
, 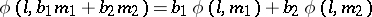 ;
; 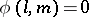 for all
for all 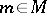 implies
implies 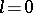 ;
; 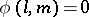 for all
for all 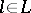 implies
implies 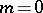 .
.
The weak topology on 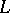 defined by the dual pair
defined by the dual pair 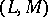 (given a topology on
(given a topology on 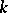 ) is the weakest topology such that all the functionals
) is the weakest topology such that all the functionals 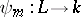 ,
, 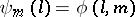 , are continuous. More precisely, if
, are continuous. More precisely, if 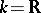 or
or 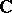 with the usual topology, this defines the weak topology on
with the usual topology, this defines the weak topology on 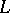 (and
(and 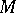 ). If
). If 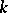 is an arbitrary field with the discrete topology, this defines the so-called linear weak topology.
is an arbitrary field with the discrete topology, this defines the so-called linear weak topology.
Let 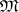 be a collection of bounded subsets of
be a collection of bounded subsets of 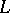 (for the weak topology, i.e. every
(for the weak topology, i.e. every 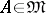 is weakly bounded, meaning that for every open subset
is weakly bounded, meaning that for every open subset 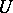 of
of  in the weak topology on
in the weak topology on 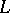 there is a
there is a 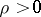 such that
such that  ). The topology
). The topology 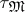 on
on 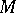 is defined by the system of semi-norms
is defined by the system of semi-norms 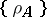 ,
, 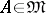 , where
, where 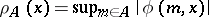 (cf. Semi-norm). This topology is locally convex if and only if
(cf. Semi-norm). This topology is locally convex if and only if 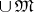 is a total set, i.e. it generates (in
is a total set, i.e. it generates (in 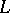 as a vector space) all of
as a vector space) all of  . The topology
. The topology  is called the topology of uniform convergence on the sets of
is called the topology of uniform convergence on the sets of 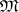 .
.
The finest topology on 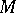 which can be defined in terms of the dual pairs
which can be defined in terms of the dual pairs 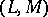 is the topology of uniform convergence on weakly bounded subsets of
is the topology of uniform convergence on weakly bounded subsets of 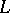 . This is the topology
. This is the topology 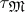 where
where 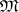 is the collection of all weakly bounded subsets of
is the collection of all weakly bounded subsets of 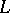 , and it is called the strong topology on
, and it is called the strong topology on 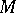 , for brevity.
, for brevity.
References
| [a1] | G. Köthe, "Topological vector spaces" , 1 , Springer (1969) |
Strong topology. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Strong_topology&oldid=48878