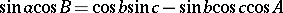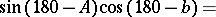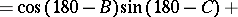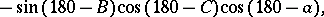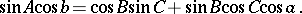The mathematical discipline that studies the interdependence of the sides and angles of spherical triangles (see Spherical geometry). Let 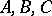 be the angles and let
be the angles and let 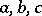 be the opposite sides of a spherical triangle
be the opposite sides of a spherical triangle  . The angles and sides of the spherical triangle are related by the following basic formulas of spherical trigonometry:
. The angles and sides of the spherical triangle are related by the following basic formulas of spherical trigonometry:
 | (1) |
(the sine theorem);
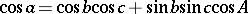 | (2) |
(the cosine theorem for sides);
(the cosine theorem for angles);
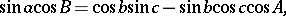 | (3) |
(the formulas linking five elements). In these formulas, the sides 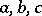 are measured by the corresponding central angles, and the lengths of these sides are equal respectively to
are measured by the corresponding central angles, and the lengths of these sides are equal respectively to 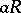 ,
, 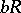 ,
, 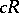 , where
, where 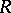 is the radius of the sphere. By changing the notations of the angles and sides according to the circular permutation:
is the radius of the sphere. By changing the notations of the angles and sides according to the circular permutation: 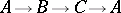
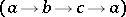 it is possible to write down other formulas of spherical trigonometry, analogous to those shown. The formulas of spherical trigonometry make it possible to determine any three elements of the spherical triangle from the other three.
it is possible to write down other formulas of spherical trigonometry, analogous to those shown. The formulas of spherical trigonometry make it possible to determine any three elements of the spherical triangle from the other three.
In order to find a spherical triangle by means of two given sides  and the angle
and the angle 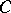 between them, and by means of two given angles
between them, and by means of two given angles 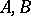 and the side
and the side  between them, the following formulas are used (Napier analogues):
between them, the following formulas are used (Napier analogues):
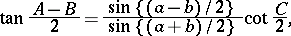 | (4) |
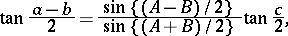 | (5) |
For right-angled spherical triangles (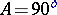 ,
,  is the hypotenuse,
is the hypotenuse, 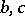 are the other two sides), the formulas are simplified, for example:
are the other two sides), the formulas are simplified, for example:
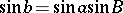 | (1prm) |
(the sine theorem);
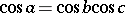 | (2prm) |
(Pythagoras' spherical theorem);
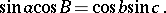 | (3prm) |
In solving problems, the following Delambre formulas, which link all six elements of a spherical triangle, are useful:
For references, see Spherical geometry.
The "analogue" in "Napier analogues" is an old-fashioned word for "proportion" .
From a relation between the elements of a spherical triangle  with sides
with sides  , a second relation can be derived by replacing every element by its supplement and at the same time replacing small letters by the corresponding capitals. An example:
, a second relation can be derived by replacing every element by its supplement and at the same time replacing small letters by the corresponding capitals. An example:
yields
i.e.
Delambre's formulas are also called Gauss' formulas (or Gauss analogues).
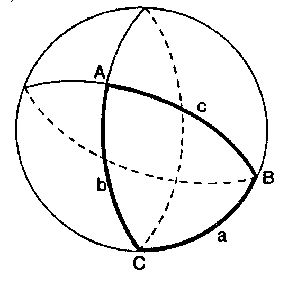
Figure: s086730a
References
| [a1] | H. Flanders, J. Price, "Trigonometry" , Acad. Press (1975) |
| [a2] | G. Hessenberg, H. Kneser, "Ebene und Sphaerische Trigonometrie" , de Gruyter (1957) |
| [a3] | W.A. Granville, P.F. Smith, J.S. Mikesh, "Spherical trigonometry" , Ginn (1943) |
| [a4] | W. Lietzmann, "Elementare Kugelgeometrie" , Vandenhoeck & Ruprecht (1949) |
| [a5] | M. Berger, "Geometry" , II , Springer (1989) |
| [a6] | B.A. [B.A. Rozenfel'd] Rosenfel'd, "A history of non-euclidean geometry" , Springer (1988) (Translated from Russian) |
| [a7] | J.D.H. Donnay, "Spherical trigonometry after the Cesàro method" (1945) |
How to Cite This Entry:
Spherical trigonometry. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Spherical_trigonometry&oldid=48779
This article was adapted from an original article by V.I. Bityutskov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098.
See original article be the angles and let
be the angles and let  be the opposite sides of a spherical triangle
be the opposite sides of a spherical triangle  . The angles and sides of the spherical triangle are related by the following basic formulas of spherical trigonometry:
. The angles and sides of the spherical triangle are related by the following basic formulas of spherical trigonometry:

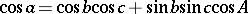
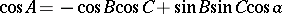
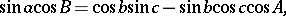
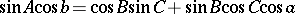
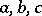 are measured by the corresponding central angles, and the lengths of these sides are equal respectively to
are measured by the corresponding central angles, and the lengths of these sides are equal respectively to 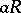 ,
, 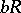 ,
, 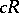 , where
, where 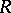 is the radius of the sphere. By changing the notations of the angles and sides according to the circular permutation:
is the radius of the sphere. By changing the notations of the angles and sides according to the circular permutation: 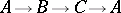
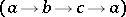 it is possible to write down other formulas of spherical trigonometry, analogous to those shown. The formulas of spherical trigonometry make it possible to determine any three elements of the spherical triangle from the other three.
it is possible to write down other formulas of spherical trigonometry, analogous to those shown. The formulas of spherical trigonometry make it possible to determine any three elements of the spherical triangle from the other three.
 and the angle
and the angle 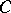 between them, and by means of two given angles
between them, and by means of two given angles 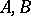 and the side
and the side  between them, the following formulas are used (Napier analogues):
between them, the following formulas are used (Napier analogues):
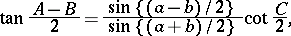
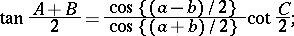
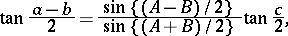
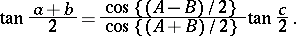
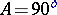 ,
,  is the hypotenuse,
is the hypotenuse, 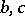 are the other two sides), the formulas are simplified, for example:
are the other two sides), the formulas are simplified, for example:
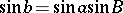
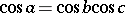
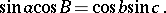
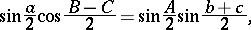
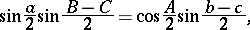

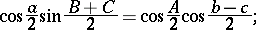
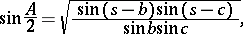

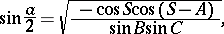
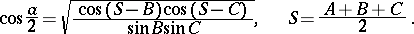
 with sides
with sides  , a second relation can be derived by replacing every element by its supplement and at the same time replacing small letters by the corresponding capitals. An example:
, a second relation can be derived by replacing every element by its supplement and at the same time replacing small letters by the corresponding capitals. An example: