Spectrum of an operator

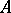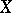The set of complex numbers for which the operator does not have an everywhere-defined bounded inverse. Here, is a linear operator on a complex Banach space and is the identity operator on . If is not closed on , then 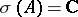, and therefore one usually considers spectra of closed operators (the spectrum of the closure of an operator for operators admitting a closure is sometimes called the closure spectrum).

If is either non-injective or non-surjective, then 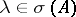. In the first case 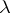is called an eigenvalue of 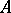; the set 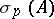of eigenvalues is called the point spectrum. In the second case 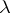is called a point of the continuous spectrum 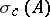or the residual spectrum 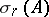, depending on whether the subspace is dense in 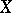or not.

There are also other classifications of the points of a spectrum. For example, 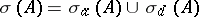, where 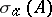consists of approximate eigenvalues ( if there are 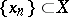with 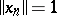such that 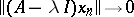), and

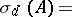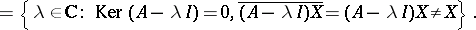Note that , and so 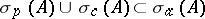. In perturbation theory, use is made of the limit spectrum 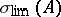, which consists of the limit points of 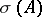and the isolated eigenvalues of infinite multiplicity, of the Weyl spectrum, which is equal to the intersection of the spectra of all compact perturbations, etc.

If is a bounded operator, then 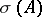is compact and non-empty (in this case coincides with the spectrum of the element 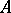of the Banach algebra 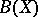, cf. Spectrum of an element); in general one can only say that 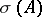is closed in . On the set 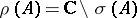one can define the analytic 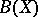-valued function 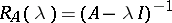, called the resolvent of 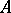( 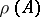is called the resolvent set). With the help of resolvents a functional calculus for 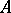is built on functions analytic in a neighbourhood of :

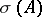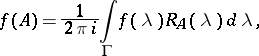where is a contour enclosing (the unboundedness of 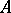imposes restrictions on the choice of 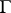). Further conditions on the geometry of the spectrum and on the asymptotics of the resolvent enables one to extend this calculus.

The spectra of operator functions are defined by the formula

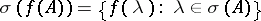(the spectral mapping theorem). The spectrum 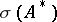of the adjoint operator coincides with 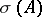when 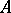is bounded; in general, .

If 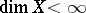, then 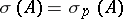, and 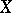decomposes into the direct sum of subspaces invariant under 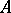, on each of which 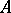induces an operator with one-point spectrum. Spectral theory of operators is concerned with finding infinite-dimensional analogues for this decomposition. See also Spectral analysis; Spectral synthesis; Spectral operator; Spectral decomposition of a linear operator.

References

[1]	N. Dunford, J.T. Schwartz, "Linear operators. General theory" , 1 , Interscience (1958)
[2]	T. Kato, "Perturbation theory for linear operators" , Springer (1980)

References

[a1]	A.E. Taylor, D.C. Lay, "Introduction to functional analysis" , Wiley (1980)
[a2]	F. Riesz, B. Szökefalvi-Nagy, "Functional analysis" , F. Ungar (1955) (Translated from French)