Spectral operator

spectral measure

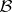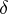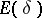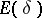A bounded linear operator mapping a Banach space into itself and such that for the -algebra of Borel subsets in the plane there is a resolution of the identity with the following properties: 1) for any the projector reduces 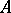, that is, 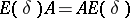and the spectrum 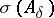lies in 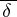, where 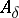is the restriction of 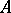to the invariant subspace ; 2) the mapping 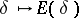is a homeomorphism of 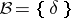into the Boolean algebra 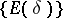; 3) all projectors 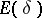are bounded, that is, , 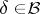; and 4) the resolution of the identity is countably additive in the strong topology of 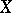, that is, for any and any sequence 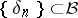of pairwise disjoint sets,

The concept of a spectral operator can be generalized to the case of closed unbounded operators. In 1), the additional requirement is then that the inclusion 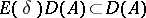holds, where 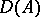is the domain of definition of 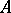, and 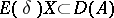for bounded .

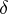All linear operators on a finite-dimensional space and all self-adjoint and normal operators on a Hilbert space are spectral operators. For example, the operator

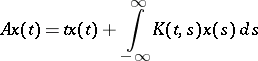on 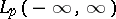, , 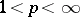is spectral on

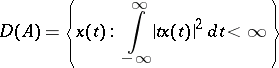if the kernel 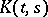is the Fourier transform of a Borel measure 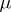on the plane of total variation and is such that

are bounded linear operators on .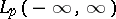

Spectral operators have many important properties, such as:

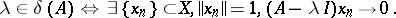If is separable, the point and residual spectra of are at most countable.

References

[1]	N. Dunford, J.T. Schwartz, "Linear operators. Spectral operators" , 3 , Interscience (1971)
[2]	N. Dunford, "A survey of the theory of spectral operators" Bull. Amer. Math. Soc. , 64 (1958) pp. 217–274