Skorokhod topology
A topological structure (topology) on the space 
of right-continuous functions on 
having limits to the left at each 
, introduced by A.V. Skorokhod [a4] as an alternative to the topology of uniform convergence in order to study the convergence in distribution of stochastic processes with jumps.
Let 
be the class of strictly increasing, continuous mappings of 
onto itself. For 
one defines
 |
The following distance, introduced by P. Billingsley [a1], induces the Skorokhod topology and makes 
a complete separable metric space:
 |
An important property is that the Borel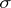 
-algebra associated with this topology coincides with the projection 
-algebra.
The Skorokhod topology on the space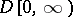 
of right-continuous functions on 
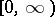having limits to the left can be defined by requiring the convergence in the Skorokhod metric on each compact interval 
, 
.
Applying Prokhorov's theorem [a3] to the complete separable metric space 
yields that a sequence 
of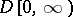 
-valued random variables (cf. Random variable) converges in distribution if and only if their finite-dimensional distributions converge and the laws of 
are tight (for every 
there exists a compact set 
such that 
for all 
). Useful criteria for weak convergence can be deduced from this result and from the characterization of compact sets in 
(see [a1]).
Complete separable distances on the space 
of functions with possible jumps on an arbitrary parameter set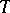 
are introduced in [a5], and for 
these distances have been applied to obtain criteria for the convergence in law of multi-parameter stochastic processes.
References
| [a1] | P. Billingsley, "Convergence of probability measures" , Wiley (1968) |
| [a2] | D. Pollard, "Convergence of stochastic processes" , Springer (1984) |
| [a3] | Y.V. Prokhorov, "Convergence of random processes and limit theorems in probability theory" Th. Probab. Appl. , 1 (1956) pp. 157–214 |
| [a4] | A.V. Skorokhod, "Limit theorems for stochastic processes" Th. Probab. Appl. , 1 (1956) pp. 261–290 |
| [a5] | M.L. Straf, "Weak convergence of stochastic processes with several parameters" , Proc. Sixth Berkeley Symp. Math. Stat. and Prob. (1972) pp. 187–221 |
Skorokhod topology. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Skorokhod_topology&oldid=48733