Self-adjoint operator
Hermitian operator
A linear operator  defined on a linear everywhere-dense set
defined on a linear everywhere-dense set  in a Hilbert space
in a Hilbert space  and coinciding with its adjoint operator
and coinciding with its adjoint operator  , that is, such that
, that is, such that  and
and
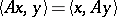 | (*) |
for every  . Every self-adjoint operator is closed and cannot be extended with the preservation of (*) to a linear manifold wider than
. Every self-adjoint operator is closed and cannot be extended with the preservation of (*) to a linear manifold wider than  ; in view of this a self-adjoint operator is also called hypermaximal. Therefore, if
; in view of this a self-adjoint operator is also called hypermaximal. Therefore, if  is a bounded self-adjoint operator, then it is defined on the whole of
is a bounded self-adjoint operator, then it is defined on the whole of 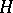 .
.
Every self-adjoint operator uniquely determines a resolution of the identity  ,
, 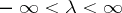 ; the following integral representation holds:
; the following integral representation holds:
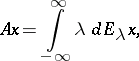 |
where the integral is understood as the strong limit of the integral sums for each 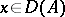 , and
, and
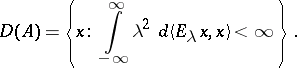 |
The spectrum of a self-adjoint operator is non-empty and lies on the real line. The quadratic form 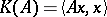 generated by a self-adjoint operator
generated by a self-adjoint operator 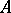 is real, and this enables one to introduce the concept of a positive operator.
is real, and this enables one to introduce the concept of a positive operator.
Many boundary value problems of mathematical physics are described by means of self-adjoint operators.
References
| [1] | L.A. Lyusternik, V.I. Sobolev, "Elements of functional analysis" , Wiley (1974) (Translated from Russian) |
| [2] | N.I. Akhiezer, I.M. Glazman, "Theory of linear operators in Hilbert space" , 1–2 , Pitman (1981) (Translated from Russian) |
| [3] | F. Riesz, B. Szökefalvi-Nagy, "Functional analysis" , F. Ungar (1955) (Translated from French) |
Comments
Cf. also Hermitian operator; Symmetric operator; Self-adjoint linear transformation.
Self-adjoint operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Self-adjoint_operator&oldid=48649