Saddle at infinity
singular saddle point
A type of arrangement of the trajectories of a dynamical system. A dynamical system 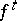 (or,
(or, 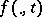 , see [1]) defined on
, see [1]) defined on 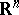 is said to have a saddle at infinity if there are points
is said to have a saddle at infinity if there are points 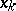 and numbers
and numbers 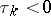 and
and 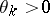 ,
, 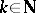 , such that the sequences
, such that the sequences
 |
are convergent and 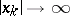 as
as 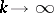 . This definition, which is due to V.V. Nemytskii, was generalized by M.V. Bebutov to dynamical systems defined on an arbitrary metric space; here the condition "xk∞ as k∞" is replaced by "the sequence xkkN does not contain any convergent subsequence" .
. This definition, which is due to V.V. Nemytskii, was generalized by M.V. Bebutov to dynamical systems defined on an arbitrary metric space; here the condition "xk∞ as k∞" is replaced by "the sequence xkkN does not contain any convergent subsequence" .
The absence of a saddle at infinity is a necessary condition for the possibility of global rectification of the dynamical system (see Complete instability). For a completely-unstable dynamical system defined on a metric space not to have a saddle at infinity it is necessary and sufficient that the quotient space of the dynamical system be Hausdorff.
References
| [1] | V.V. Nemytskii, V.V. Stepanov, "Qualitative theory of differential equations" , Princeton Univ. Press (1960) (Translated from Russian) |
Comments
The "quotient space of a dynamical systemquotient space of a dynamical system" is usually called its orbit space.
Saddle at infinity. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Saddle_at_infinity&oldid=48602